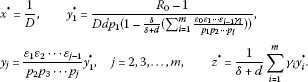Global dynamics in a class of discrete-time epidemic models with disease courses

Abstract

In this paper, a class of discrete SIRS epidemic models with disease courses is studied. The basic reproduction number $R_0$ is computed. The main results on the permanence and extinction of the disease are established. That is, the disease-free equilibrium is globally attractive if $R_0<1$, and there exists a unique endemic equilibrium and the disease is also permanent if $R_0>1$.

MSC:39A30, 92D30.

1 Introduction

In recent years, more and more attention has been paid to the discrete-time epidemic models. There are several reasons for that. Firstly, since the statistic data about a disease is collected by day, week, month or year, it is more direct, more convenient and more accurate to describe the disease by using the discrete-time models than the continuous-time models; secondly, the discrete-time models have more wealthy dynamical behaviors; for example, the single-species discrete-time models have bifurcations, chaos and other more complex dynamical behaviors.

For a discrete-time epidemic model, we see that at the present time, the main research subjects are the computation of the basic reproduction number, the local and global stability of the disease-free equilibrium and endemic equilibrium, the extinction, persistence and permanence of the disease, and the bifurcations, chaos and more complex dynamical behaviors of the model, etc. Many important and interesting results can be found in articles [1–24] and the references cited therein.

In [4], the next generation matrix approach for calculating the basic reproduction number is summarized for discrete-time epidemic models. As applications, six disease models have been developed for the study of two emerging wildlife diseases: hantavirus in rodents and chytridiomycosis in amphibians. The comparison of deterministic and stochastic SIS and SIR type epidemic models in discrete time is discussed in [3]. In [8, 9], the discrete-time SIS type epidemic models with periodic environment and with disease-induced mortality in density-dependence, respectively, are investigated. In [11], Izzo and Vecchio proposed an implicit nonlinear system of difference equations which represents the discrete counterpart of a large class of continuous models concerning the dynamics of an infection in an organism or in a host population. They also studied the limiting behavior of the discrete model and derived the basic reproduction number. Izzo, Muroya and Vecchio in [10] proved the globally asymptotic stability of the disease-free equilibrium for a general discrete-time model of population dynamics in the presence of an infection. For the discrete epidemic model with immigration of infectives, by adopting the means of the nonstandard discretization method from continuous epidemic, Jang and Eiaydi in [12] studied the globally asymptotic stability of the disease-free equilibrium, the locally asymptotic stability of the endemic equilibrium and the strong persistence of the susceptible class. Li and Wang in [15] discussed a SIS type discrete epidemic model with stage structure, where Beverton-Holt type and Richer type recruitment rates were considered, the global stability of the disease-free equilibrium and the dynamical complexity were investigated. In [17], the sufficient and necessary conditions for the global stability of the endemic equilibrium were established for a discrete epidemic model for the disease with immunity and latency in a heterogeneous host population. In [19], the bifurcations and chaos were proved in a discrete epidemic model with nonlinear incidence rates. The permanence and extinction are investigated in [20–22] for a class of discrete SIRS and SEIRS type epidemic models with time delays. In [24], a discrete mathematical model is formulated to investigate the transmission and control of SARS in China, where the basic reproductive number is obtained as a threshold to determine the asymptotic behavior of the model. Particularly, in [18] the authors studied the following class of disease epidemic models with the spread of an infection in a host population:

The global stability of disease-free equilibrium and endemic equilibrium and the permanence of the disease were obtained.

However, we know that many diseases have different disease courses, for example, tuberculosis, syphilis, AIDS, etc. Therefore, taking into account the epidemic models with disease courses is very important since disease pathogen bacteria with different course may have different reproduction and survival capacities, which indirectly influences the population growth. Under a different disease course, the transmission rate, the mortality and other vital parameters will be different [25–27].

Motivated by the above results, in this paper, we consider a class of discrete-time epidemic models with disease courses. We divide the total population into $m+2$ subgroups according to m disease courses. Let $x(n)$ be the number of susceptible individuals at the n th generation, $y_j(n)$ ($j=1,2,\dots ,m$) denote the number of infectious individuals who are in the j th course of a disease at n th generation, and let $z(t)$ denote the number of recovered individuals at the n th generation. We introduce the following assumptions.

• (1) The susceptible x has a constant input rate Λ and a natural death rate d.

• (2) The susceptible individuals of the $(n+1)$th generation are only infected by the infectious individuals of the n th generation, and ${\beta }_j$ is the constant transmission coefficient of which the susceptible is infected by compartment $y_j$.

• (3) After a susceptible individual contacts infectives and is infected, he/she will firstly enter compartment $y_1$, and then turn into compartments $y_2,y_3,\dots$ , finally into compartment $y_m$.

• (4) The infectious $y_j$ in the j th disease course admits the constant natural death rate d, the constant death rate induced by disease ${\alpha }_j$, the constant recovery rate ${\gamma }_j$ and the constant transmit rate ${\epsilon }_j$ from compartment $y_i$ to $y_{i+1}$.

• (5) The recovered z admits the constant natural death rate d, does not have permanent immunity, hence there is a constant transfer rate δ from the recovered class back to the susceptible class.

Base on the above assumptions, a class of discrete-time epidemic dynamical models with m disease courses can be established as follows:

$$\{\begin{array}{c}x(n+1)=\mathrm{\Lambda }+(1-d)x(n)+\delta z(n)-{\sum }_{i=1}^m{\beta }_i{y}_i(n)x(n+1),\hfill \\ y_1(n+1)=(1-p_1)y_1(n)+{\sum }_{i=1}^m{\beta }_i{y}_i(n)x(n+1),\hfill \\ y_j(n+1)=(1-p_j)y_j(n)+{\epsilon }_{j-1}{y}_{j-1}(n),j=2,3,\dots ,m,\hfill \\ z(n+1)=(1-\delta -d)z(n)+{\sum }_{i=1}^m{\gamma }_i{y}_i(n),\hfill \end{array}$$

(1)

where $p_i=d+{\epsilon }_i+{\alpha }_i+{\gamma }_i$, $i=1,2,\dots ,m-1$, $p_m=d+{\alpha }_m+{\gamma }_m$. For model (1), we always assume that the following basic hypotheses hold.

(H₁) For each $i=1,2,\dots ,m$, $d>0$, ${\epsilon }_i>0$, ${\alpha }_i\ge 0$, ${\beta }_i\ge 0$, ${\beta }_m>0$, ${\gamma }_i>0$, $\delta \ge 0$, $0<p_i<1$ and $0<\delta +d<1$.

(H₂) Any solution of model (1) satisfies the following initial conditions:

$$x(0)>0,y_1(0)>0,z(0)>0,y_j(0)>0,j=2,3,\dots ,m.$$

Remark 1 For model (1), we can easily see that when ${\beta }_1>0$ then model (1) describes a discrete SIRS type epidemic model with disease courses, where $y_i(n)$ ($i=1,2,\dots ,m$) denotes the number of infectious individuals in the i th course of the disease; and when ${\beta }_1=0$ then model (1) describes a discrete SEIRS type epidemic model with disease courses, where $y_1(n)$ is exposed and $y_i(n)$ ($i=2,3,\dots ,m$) denotes the number of infectious individuals in the i th course of the disease.

Remark 2 In model (1), based on the above assumption (1), we know that the disease incidence term is denoted by ${\sum }_{i=1}^m{\beta }_i{y}_i(n)x(n+1)$. This makes $x(n+1)$, i.e., the susceptible number of the $(n+1)$th generation, appear on both sides of the first equation. The reason for the above arguments is based on two considerations. On the one hand, it is influenced by the works given in [11, 18]; on the other hand, for the sake of convenience for mathematical analysis, especially, the positivity of solutions in model (1).

In this paper, by developing the methods given in [10, 11, 18], we will give the explicit expression of the basic reproduction number $R_0$. The criteria on the permanence and extinction of the disease will be established. That is, the disease-free equilibrium is globally attractive if $R_0<1$, and there exists a unique endemic equilibrium and the disease is also permanent if $R_0>1$.

This paper is organized as follows. In Section 2, as preliminaries we will give several lemmas which will be used in the proofs of the main results. In Section 3, the basic reproduction number is calculated, the existence on the disease-free equilibrium and endemic equilibrium is given and the theorem on the globally asymptotic stability of the disease-free equilibrium is stated and proved. In Section 4, we will obtain the permanence of the disease. Conclusions are presented in the last section.

2 Preliminaries

Let k be any positive integer, we denote $R_{+}^k=\{(x_1,x_2,\dots ,x_k):x_i\ge 0,i=1,2,\dots ,k\}$. For any sequence $\{f(n)\}$, we define

$$\overline{f}=\underset{n\to \mathrm{\infty }}{lim\hspace{0.17em}sup}f(n),\underline{f}=\underset{n\to \mathrm{\infty }}{lim\hspace{0.17em}inf}f(n).$$

Firstly, on the positivity of solutions of model (1), we have the following result.

Lemma 1 For any solution $(x(n),y_1(n),y_2(n),\dots ,y_m(n),z(n))$ of model (1), it holds that $x(n)>0$, $y_j(n)>0$, $z(n)>0$ ($j=1,2,\dots ,m$) for all $n>0$.

Proof From model (1), we can easily obtain

and

$$z(1)=(1-\delta -d)z(0)+\sum _{i=1}^m{\gamma }_i{y}_i(0)>0.$$

Assume that $x(n)>0$, $y_i(n)>0$ ($i=1,2,\dots ,m$) and $z(n)>0$, then we further have

and

$$z(n+1)=(1-\delta -d)z(n)+\sum _{i=1}^m{\gamma }_i{y}_i(n)>0.$$

Therefore, by using the induction, we get $x(n)>0$, $y_j(n)>0$, $z(n)>0$ for all $n>0$ and $j=1,2,\dots ,m$. This completes the proof. □

Lemma 2 For any solution $(x(n),y_1(n),y_2(n),\dots ,y_m(n),z(n))$ of model (1), it follows that

$$\overline{x}\le \frac{\mathrm{\Lambda }}{d},{\overline{y}}_j\le \frac{\mathrm{\Lambda }}{d},\overline{z}\le \frac{\mathrm{\Lambda }}{d},j=1,2,\dots ,m.$$

(2)

Proof

Let

$$N(n)=x(n)+\sum _{j=1}^m{y}_j(n)+z(n),$$

(3)

then we have

$$\begin{array}{rcl}N(n+1)& =& \mathrm{\Lambda }+(1-d)x(n)+\sum _{i=1}^m(1-d-{\alpha }_i)y_i(n)+(1-d)z(n)\\ <& \mathrm{\Lambda }+(1-d)x(n)+\sum _{i=1}^m(1-d)y_i(n)+(1-d)z(n)\\ =& \mathrm{\Lambda }+(1-d)N(n).\end{array}$$

By using the induction, we can obtain the following inequality:

$$N(n)<\frac{\mathrm{\Lambda }}{d}[1-{(1-d)}^n]+{(1-d)}^{n}N(0),$$

from which we have

$$\overline{N}=\underset{n\to \mathrm{\infty }}{lim\hspace{0.17em}sup}N(n)\le \frac{\mathrm{\Lambda }}{d}.$$

From this, we finally have

$$\overline{x}\le \frac{\mathrm{\Lambda }}{d},{\overline{y}}_j\le \frac{\mathrm{\Lambda }}{d},\overline{z}\le \frac{\mathrm{\Lambda }}{d},j=1,2,\dots ,m.$$

This completes the proof. □

On the weak permanence and permanence of the disease of model (1), we have the following definitions.

The disease in model (1) is said to be weak permanent (permanent) if there exists a constant $h>0$ such that, for any solution sequence $(x(n),y_1(n),y_2(n),\dots ,y_m(n),z(n))$ of model (1), one has

$$\underset{n\to \mathrm{\infty }}{lim\hspace{0.17em}sup}{y}_i(n)\ge h(\underset{n\to \mathrm{\infty }}{lim\hspace{0.17em}inf}{y}_i(n)\ge h),i=1,2,\dots ,m.$$

From Lemma 2, Theorem 1.1.3 and Theorem 1.10 given in [28], we can immediately obtain the following result.

Lemma 3 If the disease in model (1) is weak permanent, then it also is permanent.

Similar to Lemma 2.3 in [11] and Lemma 5 in [18], we have the following result.

Lemma 4 For any solution $(x(n),y_1(n),y_2(n),\dots ,y_m(n),z(n))$ of model (1), the following inequalities hold:

(4)

(5)

Meanwhile, we also have

$$D\underline{x}\sum _{i=1}^m{\beta }_i{\underline{y}}_i\le \sum _{i=1}^m{\beta }_i{\underline{y}}_i\le \sum _{i=1}^m{\beta }_i{\overline{y}}_i\le D\overline{x}\sum _{i=1}^m{\beta }_i{\overline{y}}_i,$$

(6)

where

$$D=\sum _{j=1}^m\frac{{\epsilon }_0{\epsilon }_1\cdots {\epsilon }_{j-1}{\beta }_j}{p_1{p}_2\cdots p_j},{\epsilon }_0=1.$$

Proof From model (1) and Lemmas 1 and 2, we easily have that

$$\underline{x}\ge \mathrm{\Lambda }+(1-d)\underline{x}-\sum _{i=1}^m{\beta }_i{\overline{y}}_i\underline{x}\ge \mathrm{\Lambda }+(1-d)\underline{x}-\sum _{i=1}^m\frac{\mathrm{\Lambda }}{d}{\beta }_i\underline{x}$$

and then

$$\underline{x}\ge \frac{d\mathrm{\Lambda }}{d^2+{\sum }_{i=1}^m{\beta }_i\mathrm{\Lambda }}>0.$$

From the third equation of model (1), we directly have

$$y_j(n+1)\ge {\epsilon }_{j-1}{y}_{j-1}(n),j=2,3,\dots ,m,n=1,2,\dots .$$

Hence, we immediately obtain that ${\overline{y}}_j\ge {\epsilon }_{j-1}{\overline{y}}_{j-1}$ for $j=2,3,\dots ,m$.

Considering the second equation of model (1), we can obtain the following inequality:

$${\overline{y}}_1=\underset{n\to \mathrm{\infty }}{lim\hspace{0.17em}sup}\{(1-p_1)y_1(n)+\sum _{i=1}^m{\beta }_i{y}_i(n)x(n+1)\}\le (1-p_1){\overline{y}}_1+\sum _{i=1}^m{\beta }_i{\overline{y}}_i\overline{x}$$

and

$${\underline{y}}_1=\underset{n\to \mathrm{\infty }}{lim\hspace{0.17em}inf}\{(1-p_1)y_1(n)+\sum _{i=1}^m{\beta }_i{y}_i(n)x(n+1)\}\ge (1-p_1){\underline{y}}_1+\sum _{i=1}^m{\beta }_i{\underline{y}}_i\underline{x}.$$

Then we have

$$\frac{1}{p_1}\sum _{i=1}^m{\beta }_i{\underline{y}}_i\underline{x}\le {\underline{y}}_1\le {\overline{y}}_1\le \frac{1}{p_1}\sum _{i=1}^m{\beta }_i{\overline{y}}_i\overline{x}.$$

(7)

Similarly, from model (1), we easily obtain

$$\frac{{\epsilon }_{j-1}}{p_j}{\underline{y}}_{j-1}\le {\underline{y}}_j\le {\overline{y}}_j\le \frac{{\epsilon }_{j-1}}{p_j}{\overline{y}}_{j-1},j=2,3,\dots ,m$$

(8)

and

$$\frac{1}{\delta +d}\sum _{i=1}^m{\gamma }_i{\underline{y}}_i\le \underline{z}\le \overline{z}\le \frac{1}{\delta +d}\sum _{i=1}^m{\gamma }_i{\overline{y}}_i.$$

Hence, from (7) and (8), it can be easily proved that

$$\prod _{i=1}^j\frac{{\epsilon }_{i-1}}{p_i}\left(\sum _{i=1}^m{\beta }_i{\underline{y}}_i\underline{x}\right)\le {\underline{y}}_j\le {\overline{y}}_j\le \prod _{i=1}^j\frac{{\epsilon }_{i-1}}{p_i}\left(\sum _{i=1}^m{\beta }_i{\overline{y}}_i\overline{x}\right).$$

(9)

Hence, inequality (5) holds.

From inequalities (7), (8) and (9), it follows that

$$\sum _{i=1}^m{\beta }_i{\overline{y}}_i\le \left(\sum _{j=1}^m\frac{{\epsilon }_0{\epsilon }_1\cdots {\epsilon }_{j-1}{\beta }_j}{p_1{p}_2\cdots p_j}\right)\left(\sum _{i=1}^m{\beta }_i{\overline{y}}_i\overline{x}\right)$$

and

$$\sum _{i=1}^m{\beta }_i{\underline{y}}_i\ge \left(\sum _{j=1}^m\frac{{\epsilon }_0{\epsilon }_1\cdots {\epsilon }_{j-1}{\beta }_j}{p_1{p}_2\cdots p_j}\right)\left(\sum _{i=1}^m{\beta }_i{\underline{y}}_i\underline{x}\right).$$

Hence, inequality (6) holds. This completes the proof. □

3 Global attractivity of disease-free equilibrium

Let the constant

$$R_0=D\frac{\mathrm{\Lambda }}{d}.$$

(10)

Firstly, on the existence of disease-free equilibrium and endemic equilibrium, we have the following result.

Theorem 1 (1) Model (1) always has a disease-free equilibrium $E_0(\frac{\mathrm{\Lambda }}{d},0,\dots ,0)$.

• (2) When $R_0>1$, model (1) also has a unique endemic equilibrium $E^{\ast }(x^{\ast },y_1^{\ast },y_2^{\ast },\dots ,y_m^{\ast },z^{\ast })$, where

  

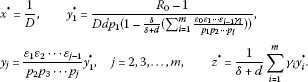
Proof The equilibrium of model (1) satisfies the following equations:

$$\{\begin{array}{c}x=\mathrm{\Lambda }+(1-d)x+\delta z-{\sum }_{i=1}^m{\beta }_i{y}_{i}x,\hfill \\ y_1=(1-p_1)y_1+{\sum }_{i=1}^m{\beta }_i{y}_{i}x,\hfill \\ y_j=(1-p_j)y_j+{\epsilon }_{j-1}{y}_{j-1},j=2,3,\dots ,m,\hfill \\ z=(1-\delta -d)z+{\sum }_{i=1}^m{\gamma }_i{y}_i.\hfill \end{array}$$

(11)

From the third equation to $(m+1)$-equation of (11), we easily obtain

$$y_j=\frac{{\epsilon }_1{\epsilon }_2\cdots {\epsilon }_{j-1}}{p_2{p}_3\cdots p_j}{y}_1,j=2,3,\dots ,m.$$

(12)

Substituting (12) into the second equation of (11), we further have

$$y_1=(\frac{{\beta }_1}{p_1}+\frac{{\epsilon }_1{\beta }_2}{p_1{p}_2}+\cdots +\frac{{\epsilon }_1{\epsilon }_2\cdots {\epsilon }_{m-1}{\beta }_m}{p_1{p}_2\cdots p_m})x{y}_1=Dx{y}_1.$$

Having solved this equality, we obtain that $y_1=0$ and $x=\frac{1}{D}$.

When $y_1=0$, then from (12) we have $y_i=0$ for $i=2,3,\dots ,m$. Further, from the first and the last equations of (11), we have $z=0$ and $x=\frac{\mathrm{\Lambda }}{d}$. This shows that model (1) has a disease-free equilibrium $E_0(\frac{\mathrm{\Lambda }}{d},0,\dots ,0)$.

When $x=\frac{1}{D}$, then from the last equation of (11), we have

$$z=\frac{1}{\delta +d}\sum _{i=1}^m{\gamma }_i{y}_i.$$

(13)

From the first equation of (11), we further obtain

$$D(\mathrm{\Lambda }+\frac{\delta }{\delta +d}\sum _{i=1}^m{\gamma }_i{y}_i)=d+\sum _{i=1}^m{\beta }_i{y}_i.$$

Substituting (12) into this equality, we further have

Hence,

$$D\mathrm{\Lambda }-d=[{\beta }_1+\sum _{i\ne 1}^m{\beta }_i\frac{{\epsilon }_1\cdots {\epsilon }_{i-1}}{p_2\cdots p_i}-D\frac{\delta }{\delta +d}({\gamma }_1+\sum _{i\ne 1}^m{\gamma }_i\frac{{\epsilon }_1\cdots {\epsilon }_{i-1}}{p_2\cdots p_i})]y_1.$$

Then, we further have

$$\begin{array}{rcl}d(R_0-1)& =& p_1{y}_1[\sum _{i=1}^m{\beta }_i\frac{{\epsilon }_0{\epsilon }_1\cdots {\epsilon }_{i-1}}{p_1{p}_2\cdots p_i}-D\frac{\delta }{\delta +d}\sum _{i=1}^m{\gamma }_i\frac{{\epsilon }_0{\epsilon }_1\cdots {\epsilon }_{i-1}}{p_1{p}_2\cdots p_i}]\\ =& p_1{y}_1[D-D\frac{\delta }{\delta +d}\sum _{i=1}^m{\gamma }_i\frac{{\epsilon }_0{\epsilon }_1\cdots {\epsilon }_{i-1}}{p_1{p}_2\cdots p_i}].\end{array}$$

Thus, we finally obtain

$$y_1=\frac{d(R_0-1)}{D{p}_1(1-\frac{\delta }{\delta +d}({\sum }_{j=1}^m\frac{{\epsilon }_0{\epsilon }_1\cdots {\epsilon }_{j-1}{\gamma }_j}{p_1{p}_2\cdots p_j}))}.$$

(14)

Since ${\epsilon }_i<p_i-{\gamma }_i$ for each $i=1,2,\dots ,m-1$ and ${\gamma }_m<p_m$, we have

Then we further obtain

Hence, we can infer that

$$\frac{\delta }{\delta +d}\left(\sum _{j=1}^m\frac{{\epsilon }_0{\epsilon }_1\cdots {\epsilon }_{j-1}{\gamma }_j}{p_1{p}_2\cdots p_j}\right)<1.$$

Thus, from (14) we obtain that $y_1>0$ if and only if $R_0>1$. Further, from (12) we obtain $y_i>0$ for $i=2,3,\dots ,m$. Finally, from (13), we also have $z>0$. Therefore, we prove that model (1) has a unique endemic equilibrium $E^{\ast }$. This completes the proof. □

Remark 3

Obviously, we have

$$R_0=\frac{{\beta }_1\mathrm{\Lambda }}{p_{1}d}+\frac{{\epsilon }_1{\beta }_2\mathrm{\Lambda }}{p_1{p}_{2}d}+\cdots +\frac{{\epsilon }_1\cdots {\epsilon }_{m-1}{\beta }_m\mathrm{\Lambda }}{p_1\cdots p_{m}d}.$$

The first term $\frac{{\beta }_1\mathrm{\Lambda }}{p_{1}d}$ denotes the ultimate number of the susceptible at the end of the first disease course which is infected by an infectious individual of the first disease course. The second term $\frac{{\epsilon }_1{\beta }_2\mathrm{\Lambda }}{p_1{p}_{2}d}$ denotes the ultimate number of the susceptible at the end of the second disease course which is infected by an infectious individual of the second disease course. And lastly, the final term $\frac{{\epsilon }_1\cdots {\epsilon }_{m-1}{\beta }_m\mathrm{\Lambda }}{p_1\cdots p_{m}d}$ denotes the ultimate number of the susceptible at the end of the m th disease course which is infected by an infectious individual of the m th disease course. We see that $R_0$ is the sum of these ultimate numbers. This shows that $R_0$ certainly is the basic reproduction number of model (1).

Theorem 2 If $R_0<1$, then the disease-free equilibrium $E_0$ of model (1) is globally attractive. That is, for any solution $(x(n),y_1(n),y_2(n),\dots ,y_m(n),z(n))$ of model (1), we have

$$\underset{n\to \mathrm{\infty }}{lim}x(n)=\frac{\mathrm{\Lambda }}{d},\underset{n\to \mathrm{\infty }}{lim}{y}_j(n)=0,\underset{n\to \mathrm{\infty }}{lim}z(n)=0,j=1,2,\dots ,m.$$

(15)

Proof Since $R_0=D\frac{\mathrm{\Lambda }}{d}<1$, then by inequality (6) in Lemma 4, we can obtain ${\underline{y}}_j={\overline{y}}_j=0$ ($j=1,2,\dots ,m$). In fact, if for some $j\in \{1,2,\dots ,m\}$ such that ${\overline{y}}_j>0$, then by inequality (4) in Lemma 4, we can obtain ${\overline{y}}_m>0$. Hence, ${\sum }_{j=1}^m{\beta }_j{\overline{y}}_j>0$. From inequality (6) in Lemma 4 and $R_0<1$, we have

$$\sum _{j=1}^m{\beta }_j{\overline{y}}_j\le D\overline{x}\sum _{j=1}^m{\beta }_j{\overline{y}}_j\le D\frac{\mathrm{\Lambda }}{d}\sum _{j=1}^m{\beta }_j{\overline{y}}_j<\sum _{j=1}^m{\beta }_j{\overline{y}}_j,$$

which leads to a contradiction. Hence, ${lim}_{n\to \mathrm{\infty }}{y}_j(n)=0$ ($j=1,2,\dots ,m$). Finally, from the expression of $x(n)$ and $z(n)$ of model (1), we can infer that (15) holds. This completes the proof. □

4 Permanence of disease

In this section, we mainly prove the permanence of model (1) when $R_0>1$. Firstly, we introduce several lemmas which will be used to study the permanence of model (1). Consider the following auxiliary system:

$$\{\begin{array}{c}{u}_1(n+1)=(1-p_1)u_1(n)+{\sum }_{i=1}^m{\beta }_{i}g(n+1)u_i(n),\hfill \\ u_j(n+1)=(1-p_j)u_j(n)+{\epsilon }_{j-1}{u}_{j-1}(n),j=2,3,\dots ,m,\hfill \\ u_{m+1}(n+1)=(1-\delta -d)y_{m+1}(n)+{\sum }_{i=1}^m{\gamma }_i{u}_i(n),\hfill \end{array}$$

(16)

where ${\{g(n)\}}_{n=1}^{\mathrm{\infty }}$ is a given non-negative bounded real sequence, and parameters $p_i$, ${\beta }_i$, ${\gamma }_i$, ${\epsilon }_j$, δ and d ($i=1,2,\dots ,m$, $j=1,2,\dots ,m-1$) are defined as in model (1). We have the following result.

Lemma 5 For any constants $\eta >0$ and $M>0$, there exist a constant $\xi =\xi (\eta )>0$ and an integer $T=T(M,\eta )>0$ such that for any initial time $n_0\in N_{+}$ and initial value $(u_{10},u_{20},\dots ,u_{m+1,0})\in R_{+}^{m+1}$ with $0<u_{i0}\le M$ ($i=1,2,\dots ,m+1$), if $u_1(n)\le \xi$ for all $n\ge n_0$, then we have

$$u_i(n)\le \eta \mathit{\text{for all}}n\ge n_0+T,i=1,2,\dots ,m+1,$$

where $(u_1(n),u_2(n),\dots ,u_{m+1}(n))$ is the solution of system (16) with the initial condition $(u_1(n_0),u_2(n_0),\dots ,u_{m+1}(n_0))=(u_{10},u_{20},\dots ,u_{m+1,0})$.

Proof Firstly, we consider the last equation of system (16)

$$u_{m+1}(n+1)=(1-\delta -d)u_{m+1}(n)+\sum _{i=1}^m{\gamma }_i{u}_i(n).$$

We can obtain that for any constants ${\eta }_1>0$ and $M>0$, there exist ${\eta }_{m+1}>0$ and $T_{m+1}>0$, with

$${\eta }_{m+1}=\frac{(\delta +d){\eta }_1}{2m}<{\eta }_1,T_{m+1}>\frac{ln{\eta }_1-ln2M}{ln(1-\delta -d)},$$

such that for any initial time $n_{m+1}>0$ and initial value $0<u_{m+1}\le M$, if $u_i(n)\le {\eta }_{m+1}$ for all $n\ge n_{m+1}$ and $i=1,2,\dots ,m$, then we have

$$\begin{array}{rcl}{u}_{m+1}(n)& \le & {(1-\delta -d)}^{n-n_{m+1}}{u}_{m+1}(n_{m+1})\\ +(\sum _{i=1}^m{\gamma }_i{u}_i(n))\left(\sum _{j=1}^{\mathrm{\infty }}{(1-\delta -d)}^i\right)\\ \le & {(1-\delta -d)}^{n-n_{m+1}}M+\frac{\gamma m{\eta }_{m+1}}{\delta +d}\\ \le & {\eta }_1\text{for all }n\ge n_{m+1}+T_{m+1}.\end{array}$$

(17)

Consider the m th equation of system (16)

$$u_m(n+1)=(1-p_m)u_m(n)+{\epsilon }_{m-1}{u}_{m-1}(n).$$

For the above constants ${\eta }_{m+1}>0$ and $M>0$, there exist a constant ${\eta }_m>0$ and an integer $T_m>0$, with

$${\eta }_m=p_m{\eta }_{m+1}<{\eta }_{m+1},T_m>\frac{ln(1-{\epsilon }_{m-1}{\eta }_{m+1})-lnM}{ln(1-p_m)},$$

such that for any initial time $n_m>0$ and initial value $0<u_m(n_m)\le M$, if $u_{m-1}(n)\le {\eta }_m$ for all $n\ge n_m$, then we have

$$\begin{array}{rcl}{u}_m(n)& \le & {(1-p_m)}^{n-n_m}{u}_m(n_m)+{\epsilon }_{m-1}{\eta }_m\sum _{i=1}^{\mathrm{\infty }}{(1-p_m)}^i\\ \le & {(1-p_m)}^{n-n_m}M+\frac{{\epsilon }_{m-1}{\eta }_m}{p_m}\\ \le & {\eta }_{m+1}\text{for all }n\ge n_m+T_m.\end{array}$$

Further consider the $(m-1)$th equation

$$u_{m-1}(n+1)=(1-p_{m-1})u_{m-1}(n)+{\epsilon }_{m-2}{u}_{m-2}(n).$$

For the above constants ${\eta }_m$ and $M>0$, there exist a constant ${\eta }_{m-1}>0$ and an integer $T_{m-1}>0$, with

$${\eta }_{m-1}=p_{m-1}{\eta }_m<{\eta }_m,T_{m-1}=\frac{ln(1-{\epsilon }_{m-2}{\eta }_m)-lnM}{ln(1-p_{m-1})},$$

such that for any initial time $n_{m-1}>0$ and initial value $0<u_{m-1}(n_{m-1})\le M$, if $u_{m-2}(n)\le {\eta }_{m-1}$ for all $n\ge n_{m-1}$, then we have

$$\begin{array}{rcl}{u}_{m-1}(n)& \le & {(1-p_{m-1})}^{n-n_{m-1}}{u}_{m-1}(n_{m-1})+{\epsilon }_{m-2}{\eta }_{m-1}\sum _{i=1}^{\mathrm{\infty }}{(1-p_{m-1})}^i\\ \le & {(1-p_{m-1})}^{n-n_{m-1}}M+\frac{{\epsilon }_{m-2}{\eta }_{m-1}}{p_{m-1}}\\ \le & {\eta }_m\text{for all }n\ge n_{m-1}+T_{m-1}.\end{array}$$

Repeating the above process for $u_{m-2}(n),\dots ,u_2(n)$, respectively, finally we can obtain that for each $u_i(n)$ ($i=2,3,\dots ,m-2$) and for the above obtained constants ${\eta }_{i+1}>0$ and $M>0$, there exist a constant ${\eta }_i>0$ and an integer $T_i>0$, with

$${\eta }_i=p_i{\eta }_{i+1}<{\eta }_{i+1},T_i>\frac{ln(1-{\epsilon }_1{\eta }_{i+1})-lnM}{ln(1-p_i)},$$

such that for any initial time $n_i>0$ and $0<u_i(n_i)\le M$, if $u_{i-1}(n)\le {\eta }_i$ for all $n\ge n_i$, then we have

$$\begin{array}{rcl}{u}_i(n)& \le & {(1-p_i)}^{n-n_i}{u}_i(n_i)+{\epsilon }_{i-1}{\eta }_i\sum _{j=1}^{\mathrm{\infty }}{(1-p_i)}^j\\ \le & {(1-p_i)}^{n-n_i}M+\frac{{\epsilon }_{i-1}{\eta }_i}{p_i}\\ \le & {\eta }_{i+1}\text{for all }n\ge n_i+T_i.\end{array}$$

(18)

Let $T={\sum }_{i=2}^{m+1}{T}_i$. Then, from the above discussions, we obtain that for any initial time $n_0>0$ and initial value $0<u_i(n_0)\le M$ ($i=1,2,\dots ,m+1$), if $u_1(n)<{\eta }_2$ for all $n\ge n_0$, then from (18) we have

$$u_2(n)<{\eta }_3<{\eta }_1\text{for all }n\ge n_0+T_2.$$

We further have

$$u_3(n)<{\eta }_4<{\eta }_1\text{for all }n\ge n_0+T_2+T_3.$$

Lastly, from (17) we have

$$u_{m+1}(n)<{\eta }_1\text{for all }n\ge n_0+T.$$

This shows

$$u_i(n)<{\eta }_1\text{for all }n\ge n_0+T,i=1,2,\dots ,m+1.$$

This completes the proof. □

We further consider the following equation:

$$v(n+1)=\mathrm{\Lambda }+(1-d)v(n)-\delta \eta -\sum _{i=1}^m{\beta }_i\eta (\frac{\mathrm{\Lambda }}{d}+1),$$

(19)

where the parameters are assumed to be as in system (1) and $0<\eta <1$. By calculating, we obtain that equation (19) has a positive equilibrium $v^{\ast }(\eta )$ with

$$v^{\ast }(\eta )=\frac{\mathrm{\Lambda }-\delta \eta -{\sum }_{i=1}^m{\beta }_i\eta (\frac{\mathrm{\Lambda }}{d}+1)}{d}.$$

Obviously, we have

$$\underset{\eta \to 0}{lim}{v}^{\ast }(\eta )=\frac{\mathrm{\Lambda }}{d}.$$

(20)

Hence, there exists an ${\eta }_0>0$ such that when $0<\eta \le {\eta }_0$, we have

$$v^{\ast }(\eta )\le \frac{\mathrm{\Lambda }}{d}+1.$$

(21)

Therefore, we have the following result.

Lemma 6 For any constants $\epsilon >0$ and $M>0$, there exists an integer $N_0=N_0(\epsilon ,M)>0$ such that for any initial $n_0>0$ and initial value $v_0$ with $0<v_0\le M$, we have

$$|v(n;n_0,\eta )-v^{\ast }(\eta )|<\epsilon \mathit{\text{for all}}n\ge n_0+N_0\mathit{\text{and}}0\le \eta \le {\eta }_0,$$

where $v(n;n_0,\eta )$ is the solution of equation (19) with the initial condition $v(n_0,\eta )=v_0$.

Proof For any solution $v(n;n_0,\eta )$ of equation (19) with the initial condition $v(n_0,\eta )=v_0>0$, we define a function

$$V(n)=|v(n;n_0,\eta )-v^{\ast }(\eta )|,$$

then

$$\begin{array}{rcl}V(n+1)& =& |v(n+1;n_0,\eta )-v^{\ast }(\eta )|\\ =& (1-d)|v(n;n_0,\eta )-v^{\ast }(\eta )|\\ =& (1-d)V(n).\end{array}$$

From this, we easily see that for any constant ε, there exists an integer $N_0=N_0(\epsilon ,M)>0$ such that for any initial time $n_0>0$ and initial value $v_0$ with $0<v_0\le M$, when $0<\eta \le {\eta }_0$, then for all $n\ge n_0+N_0$, we have

$$V(n)\le {(1-d)}^{n-n_0}(M+\frac{\mathrm{\Lambda }}{d}+1)\le {(1-d)}^{N_0}(M+\frac{\mathrm{\Lambda }}{d}+1)<\epsilon .$$

This shows that the conclusion of Lemma 6 holds. This completes the proof. □

For any $x,y\in R^m$ with $x=(x_1,x_2,\dots ,x_m)$ and $y=(y_1,y_2,\dots ,y_m)$, if $x_i\le y_i$ ($i=1,2,\dots ,m$), then we denote $x\le y$.

Let $D\subset R^m$ and $f(n,u)=(f_1(n,u),f_2(n,u),\dots ,f_m(n,u))$ be a function defined on $n\ge 0$ and $u\in D$. If for any $u_1,u_2\in D$ with $u_1\le u_2$ we have

$$f(n,u_1)\le f(n,u_2)\text{for all }n\ge 0,$$

then the function $f(n,u)$ is said to be non-decreasing for $u\in D$.

Lemma 7 (See [29])

Let the domain $D\subset R_{+}^m$ and the function $f(n,x)$ defined on $n\ge 0$ and $x\in D$ be non-decreasing for $x\in D$. If the sequence ${\{x(n)\}}_{n=1}^{\mathrm{\infty }}\subset D$ for all $n\ge n_0$ satisfies $x(n+1)\ge f(n,y(n))$ ($x(n+1)\le f(n,y(n))$), then we have

$$x(n)\ge y(n)(x(n)\le y(n))\mathit{\text{for all}}n\ge n_0,$$

where $y(n)$ is the solution of the difference equation $y(n+1)=f(n,y(n))$ with initial value $x(n_0)\ge y(n_0)$ ($x(n_0)\le y(n_0)$).

Now, we consider the following linear autonomous difference system:

$$x(n+1)=Ax(n),$$

(22)

where A is an $m\times m$ non-negative matrix and $x(n)\in R^m$. Then we have the following result.

Lemma 8 Let $r=r(A)$ be the spectral radius of matrix A, then the following conclusions hold.

• (1) There exists an m-dimensional column vector $e={(e_1,e_2,\dots ,e_m)}^T$ with $e_i>0$ ($i=1,2,\dots ,m$) such that $x(n)={\lambda }^{n}e$ is a solution of system (22).

• (2) For any $x_0=(x_{10},x_{20},\dots ,x_{m0})$ with $x_{i0}>0$ ($i=1,2,\dots ,m$), there exist constants $a_i>0$ ($i=1,2$) such that

  $${\lambda }^{n-n_0}{a}_{1}e\le x(n)\le {\lambda }^{n-n_0}{a}_{2}e\mathit{\text{for all}}n\ge n_0,$$

where $x(n)$ is the solution of system (22) satisfying the initial condition $x(n_0)=x_0$.

Proof (1) Since λ is an eigenvalue of matrix A and matrix A is a non-negative matrix, we can obtain that there is a vector $e>0$ corresponding to the eigenvalue λ such that

$$Ae=\lambda e.$$

From $A^n=A{A}^{n-1}$, we have

$$x(n)=Ax(n-1)=A^{2}x(n-2)=\cdots =A^{n}x(0).$$

Suppose that $x(0)=e$, then

$$x(n)=A^{n}e=A^{n-1}Ae=\lambda A^{n-1}e\cdots ={\lambda }^{n-1}Ae={\lambda }^{n}e.$$

Therefore, $x(n)={\lambda }^{n}e$ is a solution of system (22).

• (2) Let $b={min}_{1\le i\le m}\{x_{i0}\}$, $B={max}_{1\le i\le m}\{x_{i0}\}$, $c={min}_{1\le i\le m}\{e_i\}$ and $C={max}_{1\le i\le m}\{e_i\}$. Further let $a_1=b/C$ and $a_2=B/c$. Then we have $a_{1}e\le x_0\le a_{2}e$. Let $x_1(n)=a_{1}e{\lambda }^{n-n_0}$ and $x_2(n)=a_{2}e{\lambda }^{n-n_0}$. Then $x_1(n)$ and $x_2(n)$ are the solutions of system (22) with initial value $x_1(n_0)=a_{1}e$ and $x_2(n_0)=a_{2}e$, respectively. Then from Lemma 7 it follows that

  $${\lambda }^{n-n_0}{a}_{1}e\le x_1(n)\le x(n)\le x_2(n)\le {\lambda }^{n-n_0}{a}_{2}e$$

for all $n\ge n_0$. □

Lemma 9 If $R_0>1$, then there exists a constant $h_1>0$ such that for any solution $(x(n),y_1(n),y_2(n),\dots ,y_m(n),z(n))$ of model (1), we have

$$\underset{n\to \mathrm{\infty }}{lim\hspace{0.17em}sup}{y}_1(n)\ge h_1.$$

(23)

Proof

Since

$$R_0=\frac{D\mathrm{\Lambda }}{d}=\sum _{i=1}^m\frac{{\epsilon }_0{\epsilon }_1\cdots {\epsilon }_{i-1}{\beta }_i}{p_1{p}_2\cdots p_i}\frac{\mathrm{\Lambda }}{d}>1,$$

then we can choose a constant ${\delta }_0$ ($0<{\delta }_0<1$) such that

$$\sum _{i=1}^m\frac{{\epsilon }_0{\epsilon }_1\cdots {\epsilon }_{i-1}{\beta }_i}{p_1{p}_2\cdots p_i}(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)>1.$$

Hence, we have

$$p_1{p}_2\cdots p_m-({\beta }_1{p}_2{p}_3\cdots p_m+{\epsilon }_1{\beta }_2{p}_3\cdots p_m+\cdots +{\epsilon }_1\cdots {\epsilon }_{m-1}{\beta }_m)(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)<0.$$

(24)

Then, from (20) and (21), there exists an ${\eta }_0$ with $0<{\eta }_0\le {\delta }_0$ such that

$$\frac{\mathrm{\Lambda }}{d}-\frac{{\delta }_0}{2}\le v^{\ast }({\eta }_0)\le \frac{\mathrm{\Lambda }}{d}+1.$$

(25)

Now, from Lemma 6, for above ${\delta }_0>0$, there exists an integer $N_0>0$ such that for any initial time $n_0>0$ and initial value $v_0$ with $0<v_0\le M_0$, where $M_0=\frac{\mathrm{\Lambda }}{d}+1$, we have

$$v(n;n_0,{\eta }_0)-v^{\ast }({\eta }_0)\ge -\frac{{\delta }_0}{2}\text{for all }n\ge n_0+N_0,$$

(26)

where $v(n;n_0,{\eta }_0)$ is the solution of equation (19) with $\eta ={\eta }_0$ and the initial condition $v(n_0,{\eta }_0)=v_0$. Hence, from (25) and (26), we have

$$v(n;n_0,{\eta }_0)\ge \frac{\mathrm{\Lambda }}{d}-{\delta }_0\text{for all }n\ge n_0+N_0.$$

(27)

Assume that $(x(n),y_1(n),\dots ,y_m(n),z(n))$ is any positive solution of model (1) with the initial condition $(x(n_0),y_1(n_0),\dots ,y_m(n_0),z(n_0))=(x_0,y_{10},\dots ,y_{m0},z_0)$. Then, from Lemma 2, for $\epsilon =1$ there exists an integer $N_1>0$ such that when $n>N_1$, we have

$$x(n)\le \frac{\mathrm{\Lambda }}{d}+1,y_j(n)\le \frac{\mathrm{\Lambda }}{d}+1,z(n)\le \frac{\mathrm{\Lambda }}{d}+1,j=1,2,\dots ,m.$$

(28)

Consider the following difference system:

$$\{\begin{array}{c}{u}_1(n+1)=(1-p_1)u_1(n)+{\sum }_{i=1}^m{\beta }_{i}x(n+1)u_i(n),\hfill \\ u_j(n+1)=(1-p_j)u_j(n)+{\epsilon }_{j-1}{u}_{j-1}(n),j=2,3,\dots ,m,\hfill \\ u_{m+1}(n+1)=(1-\delta -d)u_{m+1}(n)+{\sum }_{i=1}^m{\gamma }_i{u}_i(n),\hfill \end{array}$$

(29)

where parameters $p_i$, ${\beta }_i$, ${\epsilon }_i$, ${\gamma }_i$, δ and d in system (29) are given as in model (1). Since $(x(n),y_1(n),\dots ,y_m(n),z(n))$ is the solution of model (1), then $(y_1(n),\dots ,y_m(n),z(n))$ is the solution of system (29). From (28) and Lemma 5, for above ${\eta }_0>0$ and $M=\frac{\mathrm{\Lambda }}{d}+1$, there exist a constant ${\delta }^{\ast }>0$ and an integer $N_2>0$ with ${\delta }^{\ast }\le {\eta }_0$ such that for any initial time $n_0\ge N_1$ and initial value $(u_{10},u_{20},\dots ,u_{m+1,0})\in R_{+0}^m$, if $u_1(n)\le {\delta }^{\ast }$ for all $n\ge n_0$, then we have $u_j(n)\le {\eta }_0$ for all $n\ge n_0+N_2$ and $j=1,2,\dots ,m+1$. Hence, if $y_1(n)\le {\delta }^{\ast }$ for all $n\ge n_0$, then we have

$$y_j(n)\le {\eta }_0,z(n)\le {\eta }_0\text{for all }n\ge n_0+N_2,j=1,2,\dots ,m.$$

(30)

Now, we prove that if $R_0>1$ then

$$\underset{n\to \mathrm{\infty }}{lim\hspace{0.17em}sup}{y}_1(n)\ge {\delta }^{\ast }$$

for any positive solution $(x(n),y_1(n),\dots ,y_m(n),z(n))$ of model (1). Suppose that the conclusion is not true, then there exists a positive solution $(x(n),y_1(n),\dots ,y_m(n),z(n))$ of model (1) such that ${lim\hspace{0.17em}sup}_{n\to \mathrm{\infty }}{y}_1(n)<{\delta }^{\ast }$. Hence, there exists an integer $N_1>0$ such that $y_1(n)<{\delta }^{\ast }$ for all $n\ge N_1$. From (30) we know that for any $n_0\ge N_1>0$, there exists an integer $N_2>0$ such that

$$y_j(n)\le {\eta }_0\text{and}z(n)\le {\eta }_0\text{for all }n\ge n_0+N_2,j=1,2,\dots ,m.$$

(31)

Then, from (28) and the first equation of model (1), we obtain

$$x(n+1)\ge \mathrm{\Lambda }+(1-d)x(n)-\delta {\eta }_0-\sum _{i=1}^m{\beta }_i{\eta }_0(\frac{\mathrm{\Lambda }}{d}+1).$$

Since from Lemma 7 and (27), for any $n_1\ge n_0+N_2$, there exists an integer $N_0>0$ such that

$$x(n)\ge \frac{\mathrm{\Lambda }}{d}-{\delta }_0\text{for all }n\ge n_0+N_0+N_2,$$

(32)

then replacing (32) into the second and the third equations of model (1), we have

$$\{\begin{array}{c}{y}_1(n+1)\ge (1-p_1)y_1(n)+{\sum }_{i=1}^m{\beta }_i(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)y_i(n),\hfill \\ y_2(n+1)=(1-p_2)y_2(n)+{\epsilon }_1{y}_1(n),\hfill \\ \phantom{y_2(n+1)}\cdots \cdots \cdots \hfill \\ y_m(n+1)=(1-p_m)y_m(n)+{\epsilon }_{m-1}{y}_{m-1}(n).\hfill \end{array}$$

(33)

Next, consider the following auxiliary system:

$$\{\begin{array}{c}{w}_1(n+1)=(1-p_1)w_1(n)+{\sum }_{i=1}^m{\beta }_i(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)w_i(n),\hfill \\ w_2(n+1)=(1-p_2)w_2(n)+{\epsilon }_1{w}_1(n),\hfill \\ \phantom{y_2(n+1)}\cdots \cdots \cdots \hfill \\ w_m(n+1)=(1-p_m)w_m(n)+{\epsilon }_{m-1}{w}_{m-1}(n).\hfill \end{array}$$

(34)

Obviously, system (34) is a linear autonomous difference system and we can rewrite it as follows:

$$w(n+1)=Aw(n),$$

where $w(n)={(w_1(n),w_2(n),\dots ,w_m(n))}^T$ and

$$A=\left(\begin{array}{cccc}1-p_1+{\beta }_1(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)& {\beta }_2(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)& \cdots & {\beta }_m(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)\\ {\epsilon }_1& 1-p_2& \cdots & 0\\ 0& {\epsilon }_2& \cdots & 0\\ ⋮& ⋮& \cdots & ⋮\\ 0& 0& \cdots & 0\\ 0& 0& \cdots & 1-p_m\end{array}\right).$$

We have

$$\begin{array}{rcl}f(\mu )& =& |\mu E-A|\\ =& \left|\begin{array}{cccc}\mu -1+p_1-{\beta }_1(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)& -{\beta }_2(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)& \cdots & -{\beta }_m(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)\\ -{\epsilon }_1& \mu -1+p_2& \cdots & 0\\ 0& -{\epsilon }_2& \cdots & 0\\ ⋮& ⋮& \cdots & ⋮\\ 0& 0& \cdots & 0\\ 0& 0& \cdots & \mu -1+p_m\end{array}\right|\\ =& (\mu -1+p_1-{\beta }_1(\frac{\mathrm{\Lambda }}{d}-{\delta }_0))(\mu -1+p_2)\cdots (\mu -1+p_{m-1})\\ \times (\mu -1+p_m)-{\epsilon }_1{\beta }_2(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)(\mu -1+p_3)\cdots (\mu -1+p_{m-1})\\ \times (\mu -1+p_m)-{\epsilon }_1{\epsilon }_2{\beta }_3(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)(\mu -1+p_4)\cdots (\mu -1+p_{m-1})\\ \times (\mu -1+p_m)-\cdots -{\epsilon }_1{\epsilon }_2\cdots {\epsilon }_{m-1}{\beta }_m(\frac{\mathrm{\Lambda }}{d}-{\delta }_0).\end{array}$$

From (24), we have

$$\begin{array}{rcl}f(1)& =& (p_1-{\beta }_1(\frac{\mathrm{\Lambda }}{d}-{\delta }_0))p_2{p}_3\cdots p_m-{\epsilon }_1{\beta }_2(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)p_3\cdots p_m\\ -{\epsilon }_1{\epsilon }_2{\beta }_3(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)p_3\cdots p_m-\cdots -{\epsilon }_1\cdots {\epsilon }_{m-2}{\beta }_{m-1}(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)p_m\\ -{\epsilon }_1{\epsilon }_2\cdots {\epsilon }_{m-1}{\beta }_m(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)\\ =& p_1{p}_2\cdots p_m-({\beta }_1{p}_2{p}_3\cdots p_m+{\epsilon }_1{\beta }_2{p}_3\cdots p_m+\cdots \\ +{\epsilon }_1\cdots {\epsilon }_{m-1}{\beta }_m)(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)\\ <& 0\end{array}$$

and, for a constant $\beta >max\{{\beta }_1,{\beta }_2,\dots ,{\beta }_m\}(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)$, we further have

$$\begin{array}{rcl}f(\beta +1)& =& (\beta +p_1-{\beta }_1(\frac{\mathrm{\Lambda }}{d}-{\delta }_0))(\beta +p_2)(\beta +p_3)\cdots (\beta +p_m)\\ -{\epsilon }_1{\beta }_2(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)(\beta +p_3)\cdots (\beta +p_m)-\cdots \\ -{\epsilon }_1\cdots {\epsilon }_{m-2}{\beta }_{m-1}(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)(\beta +p_m)\\ -{\epsilon }_1{\epsilon }_2\cdots {\epsilon }_{m-1}{\beta }_m(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)\\ =& (\beta -{\beta }_1(\frac{\mathrm{\Lambda }}{d}-{\delta }_0))(\beta +p_2)\cdots (\beta +p_m)\\ +p_1\beta (\beta +p_3)\cdots (\beta +p_m)+p_1{p}_2\beta (\beta +p_4)\cdots (\beta +p_m)\\ +\cdots +p_1{p}_2\cdots p_{m-2}\beta (\beta +p_m)+p_1{p}_2\cdots p_{m-1}(\beta +p_m)\\ -{\epsilon }_1{\beta }_2(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)(\beta +p_3)\cdots (\beta +p_m)-\cdots \\ -{\epsilon }_1\cdots {\epsilon }_{m-2}{\beta }_{m-1}(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)(\beta +p_m)\\ -{\epsilon }_1{\epsilon }_2\cdots {\epsilon }_{m-1}{\beta }_m(\frac{\mathrm{\Lambda }}{d}-{\delta }_0)\\ >& (\beta -{\beta }_1(\frac{\mathrm{\Lambda }}{d}-{\delta }_0))(\beta +p_2)\cdots (\beta +p_m)\\ +p_1(\beta -{\beta }_2(\frac{\mathrm{\Lambda }}{d}-{\delta }_0))(\beta +p_3)\cdots (\beta +p_m)\\ +p_1{p}_2(\beta -{\beta }_3(\frac{\mathrm{\Lambda }}{d}-{\delta }_0))(\beta +p_4)\cdots (\beta +p_m)\\ +\cdots +p_1\cdots p_{m-2}(\beta -{\beta }_{m-1}(\frac{\mathrm{\Lambda }}{d}-{\delta }_0))(\beta +p_m)\\ +p_1{p}_2\cdots p_{m-1}(\beta -{\beta }_m(\frac{\mathrm{\Lambda }}{d}-{\delta }_0))\\ >& 0.\end{array}$$

Then, from the intermediate value theorem, there exists a constant ${\mu }^{\ast }\in (1,\beta +1)$ such that $f({\mu }^{\ast })=0$. Therefore, we can obtain $\overline{\mu }=r(A)>1$. Let $w(n)$ be the solution of system (33) with the initial condition $w(N^{\ast })=(y_1(N^{\ast }),y_2(N^{\ast }),\dots ,y_m(N^{\ast }))$, where $N^{\ast }=n_0+N_0+N_2$. Then from (33), (34) and Lemma 7, we have

$$y(n)\ge w(n)\text{for all }n\ge N^{\ast }.$$

Further, from the second part of Lemma 8, there exists a constant $a_1>0$ such that

$$w(n)\ge {\overline{\mu }}^{n-n_0-N_0-N_2}{a}_{1}e.$$

Hence, we have

$$y_1(n)\ge {\overline{\mu }}^{n-n_0-N_0-N_2}{a}_1{e}_1\ge {\overline{\mu }}^{n-n_0-N_0-N_2}{a}_{1}c.$$

From this, we obtain

$$\underset{n\to \mathrm{\infty }}{lim}{y}_1(n)=\mathrm{\infty },$$

which leads to a contradiction. This completes the proof. □

Lastly, directly from Lemma 3, Lemma 4 and Lemma 9, we can obtain the following result on the permanence of model (1).

Theorem 3 If $R_0>1$, then the disease in model (1) is permanent.

Proof In fact, from Lemma 9, we obtain that for any positive solution $(x(n),y_1(n),\dots ,y_m(n),z(n))$ of model (1),

$$\underset{n\to \mathrm{\infty }}{lim\hspace{0.17em}sup}{y}_1(n)\ge h_1>0.$$

Then, from inequality (4) in Lemma 4, we further have

$$\underset{n\to \mathrm{\infty }}{lim\hspace{0.17em}sup}{y}_j(n)\ge {\epsilon }_2{\epsilon }_3\cdots {\epsilon }_{j-1}\underset{n\to \mathrm{\infty }}{lim\hspace{0.17em}sup}{y}_1(n)\ge {\epsilon }_2{\epsilon }_3\cdots {\epsilon }_{j-1}{h}_1,j=2,3,\dots ,m.$$

Finally, from the last equation of model (1), we have

$$\underset{n\to \mathrm{\infty }}{lim\hspace{0.17em}sup}z(n)\ge \sum _{i=1}^m{\gamma }_i\underset{n\to \mathrm{\infty }}{lim\hspace{0.17em}sup}{y}_i(n)\ge \sum _{i=1}^m{\gamma }_i{\epsilon }_2{\epsilon }_3\cdots {\epsilon }_{i-1}{h}_1.$$

This completes the proof. □

5 Conclusions

In this paper, we study a class of discrete epidemic models with disease courses, that is, model (1). The basic reproduction number $R_0$ is calculated. It is shown that the global dynamics of model (1) is determined by the basic reproduction number $R_0$. If $R_0<1$, then we obtain that the disease-free equilibrium of model (1) is globally asymptotically stable. This shows that when $R_0<1$ the disease in model (1) is extinct. If $R_0>1$, then we obtain that the endemic equilibrium of model (1) exists and the disease is permanent. Clearly, our condition given in this paper is the threshold condition between the extinction and the permanence of the disease. Hence, our results obtained in this paper extend the results given in [7, 11, 18] for the discrete epidemic models.

However, it is a pity that the case of the basic reproduction number $R_0=1$ is not discussed in this paper. From the results on the case $R_0=1$ obtained in [17, 18], we can guess that when $R_0=1$ then the disease-free equilibrium of model (1) is also globally asymptotically stable. This shows that when $R_0=1$ the disease in model (1) is also extinct. The other one which is not obtained in our this paper is the global stability of the endemic equilibrium of model (1). From the results on the global stability of the endemic equilibrium obtained in [7, 17, 18], we can guess that when $R_0>1$ the endemic equilibrium of model (1) is globally stable. We will discuss these problems in our future work.