Basins of attraction of certain rational anti-competitive system of difference equations in the plane

Abstract

We investigate the global asymptotic behavior of solutions of the following anti-competitive system of rational difference equations:

$$x_{n+1}=\frac{{\gamma }_1{y}_n}{A_1+x_n},y_{n+1}=\frac{{\beta }_2{x}_n}{A_2+B_2{x}_n+y_n},n=0,1,\dots ,$$

where the parameters ${\gamma }_1$, ${\beta }_2$, $A_1$, $A_2$ and $B_2$ are positive numbers and the initial conditions $(x_0,y_0)$ are arbitrary nonnegative numbers. We find the basins of attraction of all attractors of this system, which are the equilibrium points and period-two solutions.

MSC:39A10, 39A11.

1 Introduction

A first-order system of difference equations

$$\{\begin{array}{c}{x}_{n+1}=f(x_n,y_n),\\ y_{n+1}=g(x_n,y_n),\end{array}n=0,1,\dots ,(x_0,y_0)\in \mathcal{R},$$

(1)

where $\mathcal{R}\subset {\mathbb{R}}^2$, $(f,g):\mathcal{R}\to \mathcal{R},f,g$ are continuous functions is competitive if $f(x,y)$ is non-decreasing in x and non-increasing in y, and $g(x,y)$ is non-increasing in x and non-decreasing in y.

System (1) where the functions f and g have a monotonic character opposite of the monotonic character in competitive system will be called anti-competitive.

We consider the following anti-competitive system of difference equations:

$$x_{n+1}=\frac{{\gamma }_1{y}_n}{A_1+x_n},y_{n+1}=\frac{{\beta }_2{x}_n}{A_2+B_2{x}_n+y_n},n=0,1,\dots ,$$

(2)

where the parameters $A_1$, ${\gamma }_1$, $A_2$, $B_2$ and ${\beta }_2$ are positive numbers and the initial conditions $(x_0,y_0)$ are arbitrary nonnegative numbers. In the classification of all linear fractional systems in [1], System (2) was mentioned as System (16, 39).

Competitive and cooperative systems of the form (1) were studied by many authors such as Clark and Kulenović [2], Clark, Kulenović and Selgrade [3], Hirsch and Smith [4], Kulenović and Ladas [5], Kulenović and Merino [6], Kulenović and Nurkanović [7, 8], Garić-Demirović, Kulenović and Nurkanović [9, 10], Smith [11, 12] and others.

The study of anti-competitive systems started in [13] and has advanced since then (see [14, 15]). The principal tool of the study of anti-competitive systems is the fact that the second iterate of the map associated with an anti-competitive system is a competitive map, and so the elaborate theory for such maps developed recently in [4, 16, 17] can be applied.

The main result on the global behavior of System (2) is the following theorem.

Theorem 1 (a) If ${\beta }_2{\gamma }_1\le A_1{A}_2$, then $E_0=(0,0)$ is a unique equilibrium, and the basin of attraction of this equilibrium is $\mathcal{B}(E_0)=\{(x,y):x\ge 0,y\ge 0\}$ (see Figure 1(a)).

Figure 1

Basins of attraction

(b) If ${\beta }_2{\gamma }_1-A_1{A}_2>-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)]$ and ${\beta }_2{\gamma }_1-A_1{A}_2>0$, then there exist two equilibrium points: $E_0$ which is a repeller and $E_{+}$ which is an interior saddle point, and minimal period-two solutions $A_0=(0,\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{{\gamma }_1{B}_2})$ and $B_0=(\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{A_1{B}_2},0)$ which are locally asymptotically stable. There exists a set $\mathcal{C}\subset \mathcal{R}=[0,\mathrm{\infty })\times [0,\mathrm{\infty })$ such that $E_0\in \mathcal{C}$, and ${\mathcal{W}}^s(E_{+})=\mathcal{C}\setminus E_0$ is an invariant subset of the basin of attraction of $E_{+}$. The set $\mathcal{C}$ is a graph of a strictly increasing continuous function of the first variable on an interval and separates $\mathcal{R}$ into two connected and invariant components, namely

$${\mathcal{W}}_{-}:=\{\mathbf{x}\in \mathcal{R}\mathrm{\setminus }\mathcal{C}:\mathrm{\exists }{\mathbf{x}}^{\mathrm{\prime }}\in \mathcal{C}\mathit{\text{with}}\mathbf{x}{⪯}_{se}{\mathbf{x}}^{\mathrm{\prime }}\},{\mathcal{W}}_{+}:=\{\mathbf{x}\in \mathcal{R}\mathrm{\setminus }\mathcal{C}:\mathrm{\exists }{\mathbf{x}}^{\mathrm{\prime }}\in \mathcal{C}\mathit{\text{with}}{\mathbf{x}}^{\mathrm{\prime }}{⪯}_{se}\mathbf{x}\},$$

which satisfy (see Figure 1(b)):

(i) If $(x_0,y_0)\in {\mathcal{W}}_{+}$, then

$$\underset{n\to \mathrm{\infty }}{lim}(x_{2n},y_{2n})=(\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{A_1{B}_2},0)=B_0$$

and

$$\underset{n\to \mathrm{\infty }}{lim}(x_{2n+1},y_{2n+1})=(0,\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{{\gamma }_1{B}_2})=A_0.$$

(ii) If $(x_0,y_0)\in {\mathcal{W}}_{-}$, then

$$\underset{n\to \mathrm{\infty }}{lim}(x_{2n},y_{2n})=(0,\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{{\gamma }_1{B}_2})=A_0$$

and

$$\underset{n\to \mathrm{\infty }}{lim}(x_{2n+1},y_{2n+1})=(\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{A_1{B}_2},0)=B_0.$$

(c) If $0<{\beta }_2{\gamma }_1-A_1{A}_2=-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)]$, then (see Figure 1(c))

(i) There exist two equilibrium points: $E_0$ which is a repeller and $E_{+}\in int(\mathcal{R})$ which is a non-hyperbolic, and an infinite number of minimal period-two solutions

for $x\in [0,\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{A_1{B}_2}]$, that belong to the segment of the line (15) in the first quadrant.

(ii) All minimal period-two solutions and the equilibrium $E_{+}$ are stable but not asymptotically stable.

(iii) There exists a family of strictly increasing curves ${\mathcal{C}}_{+}$, ${\mathcal{C}}_{A_x}$, ${\mathcal{C}}_{B_x}$ for $x\in (0,\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{A_1{B}_2})$ and

$${\mathcal{C}}_{A_0}=\{(x,y):x=0,y>0\},{\mathcal{C}}_{B_0}=\{(x,y):x>0,y=0\}$$

that emanate from $E_0$ and $A_x\in {\mathcal{C}}_{A_x}$, $B_x\in {\mathcal{C}}_{B_x}$ for all $x\in [0,\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{A_1{B}_2})$, such that the curves are pairwise disjoint, the union of all the curves equals ${\mathbb{R}}_{+}^2$. Solutions with initial points in ${\mathcal{C}}_{+}$ converge to $E_{+}$ and solutions with an initial point in ${\mathcal{C}}_{A_x}$ have even-indexed terms converging to $A_x$ and odd-indexed terms converging to $B_x$; solutions with an initial point in ${\mathcal{C}}_{B_x}$ have even-indexed terms converging to $B_x$ and odd-indexed terms converging to $A_x$.

(d) If $0<{\beta }_2{\gamma }_1-A_1{A}_2<-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)]$, then System (2) has two equilibrium points: $E_0$ which is a repeller and $E_{+}$ which is locally asymptotically stable, and minimal period-two solutions $A_0$ and $B_0$ which are saddle points. The basin of attraction of the equilibrium point $E_{+}$ is the set

$$\mathcal{B}(E_{+})=\{(x,y):x>0,y>0\}$$

and solutions with an initial point in $\{(x,y):x=0,y>0\}$ have even-indexed terms converging to $A_0$ and odd-indexed terms converging to $B_0$, solutions with an initial point in $\{(x,y):x>0,y=0\}$ have even-indexed terms converging to $B_0$ and odd-indexed terms converging to $A_0$ (see Figure 1(d)).

2 Preliminaries

We now give some basic notions about systems and maps in the plane of the form (1).

Consider a map $T=(f,g)$ on a set $\mathcal{R}\subset {\mathbf{R}}^2$, and let $E\in \mathcal{R}$. The point $E\in \mathcal{R}$ is called a fixed point if $T(E)=E$. An isolated fixed point is a fixed point that has a neighborhood with no other fixed points in it. A fixed point $E\in \mathcal{R}$ is an attractor if there exists a neighborhood $\mathcal{U}$ of E such that $T^n(\mathbf{x})\to E$ as $n\to \mathrm{\infty }$ for $\mathbf{x}\in \mathcal{U}$; the basin of attraction is the set of all $\mathbf{x}\in \mathcal{R}$ such that $T^n(\mathbf{x})\to E$ as $n\to \mathrm{\infty }$. A fixed point E is a global attractor on a set $\mathcal{K}$ if E is an attractor and $\mathcal{K}$ is a subset of the basin of attraction of E. If T is differentiable at a fixed point E, and if the Jacobian $J_T(E)$ has one eigenvalue with modulus less than one and a second eigenvalue with modulus greater than one, E is said to be a saddle. See [18] for additional definitions.

Here we give some basic facts about the monotone maps in the plane, see [11, 16, 17, 19]. Now, we write System (2) in the form

$$(\begin{array}{c}x\\ y\end{array}{)}_{n+1}=T(\begin{array}{c}x\\ y\end{array}{)}_n,$$

where the map T is given as

$$T:\left(\begin{array}{c}x\\ y\end{array}\right)\to \left(\begin{array}{c}\frac{{\gamma }_{1}y}{A_1+x}\\ \frac{{\beta }_{2}x}{A_2+B_{2}x+y}\end{array}\right)=\left(\begin{array}{c}f(x,y)\\ g(x,y)\end{array}\right).$$

(3)

The map T may be viewed as a monotone map if we define a partial order on ${\mathbf{R}}^2$ so that the positive cone in this new partial order is the fourth quadrant. Specifically, for $\mathbf{v}=(v_1,v_2)$, $\mathbf{w}=(w_1,w_2)\in {\mathbf{R}}^2$ we say that $\mathbf{v}⪯\mathbf{w}$ if $v_1\le w_1$ and $w_2\le v_2$. Two points $\mathbf{v},\mathbf{w}\in {\mathbf{R}}_{+}^2$ are said to be related if $\mathbf{v}⪯\mathbf{w}$ or $\mathbf{w}⪯\mathbf{v}$. Also, a strict inequality between points may be defined as $\mathbf{v}\prec \mathbf{w}$ if $\mathbf{v}⪯\mathbf{w}$ and $\mathbf{v}\ne \mathbf{w}$. A stronger inequality may be defined as $\mathbf{v}\prec \prec \mathbf{w}$ if $v_1<w_1$ and $w_2<v_2$. A map $f:int{\mathbf{R}}_{+}^2\to Int{\mathbf{R}}_{+}^2$ is strongly monotone if $\mathbf{v}\prec \mathbf{w}$ implies that $f(\mathbf{v})\prec \prec f(\mathbf{w})$ for all $\mathbf{v},\mathbf{w}\in Int{\mathbf{R}}_{+}^2$. Clearly, being related is an invariant under iteration of a strongly monotone map. Differentiable strongly monotone maps have Jacobian with constant sign configuration

$$\left[\begin{array}{cc}+& -\\ -& +\end{array}\right].$$

The mean value theorem and the convexity of ${\mathbf{R}}_{+}^2$ may be used to show that T is monotone, as in [20].

For $\mathbf{x}=(x_1,x_2)\in {\mathbb{R}}^2$, define $Q_l(\mathbf{x})$ for $l=1,\dots ,4$ to be the usual four quadrants based at x and numbered in a counterclockwise direction, for example, $Q_1(\mathbf{x})=\{\mathbf{y}=(y_1,y_2)\in {\mathbb{R}}^2:x_1\le y_1,x_2\le y_2\}$.

The following definition is from [11].

Definition 1 Let $\mathcal{S}$ be a nonempty subset of ${\mathbb{R}}^2$. A competitive map $T:\mathcal{S}\to \mathcal{S}$ is said to satisfy condition (O+) if for every x, y in $\mathcal{S}$, $T(x){⪯}_{ne}T(y)$ implies $x{⪯}_{ne}y$, and T is said to satisfy condition (O−) if for every x, y in $\mathcal{S}$, $T(x){⪯}_{ne}T(y)$ implies $y{⪯}_{ne}x$.

The following theorem was proved by de Mottoni-Schiaffino for the Poincaré map of a periodic competitive Lotka-Volterra system of differential equations. Smith generalized the proof to competitive and cooperative maps [11].

Theorem 2 Let $\mathcal{S}$ be a nonempty subset of ${\mathbb{R}}^2$. If T is a competitive map for which (O+) holds then for all $x\in \mathcal{S}$, $\{T^n(x)\}$ is eventually componentwise monotone. If the orbit of x has compact closure, then it converges to a fixed point of T. If instead (O−) holds, then for all $x\in \mathcal{S}$, $\{T^{2n}\}$ is eventually componentwise monotone. If the orbit of x has compact closure in $\mathcal{S}$, then its omega limit set is either a period-two orbit or a fixed point.

The following result is from [11], with the domain of the map specialized to be the Cartesian product of intervals of real numbers. It gives a sufficient condition for conditions (O+) and (O−).

Theorem 3 Let $\mathcal{R}\subset {\mathbb{R}}^2$ be the Cartesian product of two intervals in $\mathbb{R}$. Let $T:\mathcal{R}\to \mathcal{R}$ be a $C^1$ competitive map. If T is injective and $det{J}_T(x)>0$ for all $x\in \mathcal{R}$ then T satisfies (O+). If T is injective and $det{J}_T(x)<0$ for all $x\in \mathcal{R}$ then T satisfies (O−).

Next two results are from [17, 21].

Theorem 4 Let T be a competitive map on a rectangular region $\mathcal{R}\subset {\mathbb{R}}^2$. Let $\overline{\mathbf{x}}\in \mathcal{R}$ be a fixed point of T such that $\mathrm{\Delta }:=\mathcal{R}\cap int(Q_1(\overline{\mathbf{x}})\cup Q_3(\overline{\mathbf{x}}))$ is nonempty (i.e., $\overline{\mathbf{x}}$ is not the NW or SE vertex of $\mathcal{R}$), and T is strongly competitive on Δ. Suppose that the following statements are true.

• a. The map T has a $C^1$ extension to a neighborhood of $\overline{\mathbf{x}}$.

• b. The Jacobian matrix of T at $\overline{\mathbf{x}}$ has real eigenvalues λ, μ such that $0<|\lambda |<\mu$, where $|\lambda |<1$, and the eigenspace $E^{\lambda }$ associated with λ is not a coordinate axis.

Then there exists a curve $\mathcal{C}\subset \mathcal{R}$ through $\overline{\mathbf{x}}$ that is invariant and a subset of the basin of attraction of $\overline{\mathbf{x}}$, such that $\mathcal{C}$ is tangential to the eigenspace $E^{\lambda }$ at $\overline{\mathbf{x}}$, and $\mathcal{C}$ is the graph of a strictly increasing continuous function of the first coordinate on an interval. Any endpoints of $\mathcal{C}$ in the interior of $\mathcal{R}$ are either fixed points or minimal period-two points. In the latter case, the set of endpoints of $\mathcal{C}$ is a minimal period-two orbit of T.

Theorem 5 (Kulenović & Merino)

Let ${\mathcal{I}}_1$, ${\mathcal{I}}_2$ be intervals in $\mathbb{R}$ with endpoints $a_1$, $a_2$ and $b_1$, $b_2$ with endpoints respectively, with $a_1<a_2$ and $b_1<b_2$, where $-\mathrm{\infty }\le a_1<a_2\le \mathrm{\infty }$ and $-\mathrm{\infty }\le b_1<b_2\le \mathrm{\infty }$. Let T be a competitive map on a rectangle ${\mathcal{R}=\mathcal{I}}_1\times {\mathcal{I}}_2$ and $\overline{\mathbf{x}}\in int(\mathcal{R})$. Suppose that the following hypotheses are satisfied:

• 1. $T(int(\mathcal{R}))\subset int(\mathcal{R})$ and T is strongly competitive on $int(\mathcal{R})$.

• 2. The point $\overline{\mathbf{x}}$ is the only fixed point of T in $(Q_1(\overline{\mathbf{x}})\cup Q_3(\overline{\mathbf{x}}))\cap int(\mathcal{R})$.

• 3. The map T is continuously differentiable in a neighborhood of $\overline{\mathbf{x}}$.

• 4. At least one of the following statements is true:

• a. T has no minimal period two orbits in $(Q_1(\overline{\mathbf{x}})\cup Q_3(\overline{\mathbf{x}}))\cap int(\mathcal{R})$.

• b. $det{J}_T(\overline{\mathbf{x}})>0$ and $T(\mathbf{x})=\overline{\mathbf{x}}$ only for $\mathbf{x}=\overline{\mathbf{x}}$.

• 5. $\overline{\mathbf{x}}$ is a saddle point.

Then the following statements are true.

• (i) The stable manifold ${\mathcal{W}}^s(\overline{\mathbf{x}})$ is connected and it is the graph of a continuous increasing curve with endpoints in $\partial \mathcal{R}$. $int(\mathcal{R})$ is divided by the closure of ${\mathcal{W}}^s(\overline{\mathbf{x}})$ into two invariant connected regions ${\mathcal{W}}_{+}$ (“below the stable set”), and ${\mathcal{W}}_{-}$ (“above the stable set”), where

  

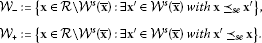
• (ii) The unstable manifold ${\mathcal{W}}^u(\overline{\mathbf{x}})$ is connected, and it is the graph of a continuous decreasing curve.

• (iii) For every $\mathbf{x}\in {\mathcal{W}}_{+}$, $T^n(\mathbf{x})$ eventually enters the interior of the invariant set $Q_4(\overline{\mathbf{x}})\cap \mathcal{R}$, and for every $\mathbf{x}\in {\mathcal{W}}_{-}$, $T^n(\mathbf{x})$ eventually enters the interior of the invariant set $Q_2(\overline{\mathbf{x}})\cap \mathcal{R}$.

• (iv) Let $\mathbf{m}\in Q_2(\overline{\mathbf{x}})$ and $\mathbf{M}\in Q_4(\overline{\mathbf{x}})$ be the endpoints of ${\mathcal{W}}^u(\overline{\mathbf{x}})$, where $\mathbf{m}{⪯}_{se}\overline{\mathbf{x}}{⪯}_{se}\mathbf{M}$. For every $\mathbf{x}\in {\mathcal{W}}_{-}$ and every $\mathbf{z}\in \mathcal{R}$ such that $\mathbf{m}{⪯}_{se}z$, there exists $m\in \mathbb{N}$ such that $T^m(\mathbf{x}){⪯}_{se}z$, and for every $\mathbf{x}\in {\mathcal{W}}_{+}$ and every $\mathbf{z}\in \mathcal{R}$ such that $\mathbf{z}{⪯}_{se}\mathbf{M}$, there exists $m\in \mathbb{N}$ such that $\mathbf{M}{⪯}_{se}{T}^m(\mathbf{x})$.

3 Linearized stability analysis

Lemma 1

• (i) If ${\beta }_2{\gamma }_1-A_1{A}_2\le 0$, then System (2) has a unique equilibrium point $E_0=(0,0)$.

• (ii) If ${\beta }_2{\gamma }_1-A_1{A}_2>0$, then System (2) has two equilibrium points $E_0$ and $E_{+}=(\overline{x},\overline{y})$, $\overline{x}>0$, $\overline{y}>0$.

Proof The equilibrium point $E(\overline{x},\overline{y})$ of System (2) satisfies the following system of equations:

$$\overline{x}=\frac{{\gamma }_1\overline{y}}{A_1+\overline{x}},\overline{y}=\frac{{\beta }_2\overline{x}}{A_2+B_2\overline{x}+\overline{y}}.$$

(4)

It is easy to see that $E_0=(0,0)$ is one equilibrium point for all values of the parameters, and $E_{+}=(\overline{x},\overline{y})$ is a positive equilibrium point if ${\beta }_2{\gamma }_1-A_1{A}_2>0$. Indeed, substituting $\overline{y}$ from the first equation in (4) in the second equation in (4), we obtain that $\overline{x}$ satisfies the following equation:

$$f(x)=x^3+(2A_1+B_2{\gamma }_1)x^2+(A_1^2+A_1{B}_2{\gamma }_1+A_2{\gamma }_1)x+{\gamma }_1(A_1{A}_2-{\beta }_2{\gamma }_1)=0.$$

(5)

By using Descartes’ theorem, we have that equation (5) has one positive equilibrium if the condition

$${\beta }_2{\gamma }_1-A_1{A}_2>0$$

(6)

is satisfied, i.e., ${\beta }_2{\gamma }_1>A_1{A}_2$. □

Theorem 6

• (i) If ${\beta }_2{\gamma }_1<A_1{A}_2$, then $E_0$ is locally asymptotically stable.

• (ii) If ${\beta }_2{\gamma }_1=A_1{A}_2$, then $E_0$ is non-hyperbolic.

• (iii) If ${\beta }_2{\gamma }_1>A_1{A}_2$, then $E_0$ is a repeller.

Proof The map T associated to System (2) is of the form (3). The Jacobian matrix of T at the equilibrium $E=(\overline{x},\overline{y})$ is

$$J_T(\overline{x},\overline{y})=\left(\begin{array}{cc}-\frac{{\gamma }_1\overline{y}}{{(A_1+\overline{x})}^2}& \frac{{\gamma }_1}{A_1+\overline{x}}\\ \frac{{\beta }_2(A_2+\overline{y})}{{(A_2+B_2\overline{x}+\overline{y})}^2}& -\frac{{\beta }_2\overline{x}}{{(A_2+B_2\overline{x}+\overline{y})}^2}\end{array}\right)$$

(7)

and

$$J_T(0,0)=\left(\begin{array}{cc}0& \frac{{\gamma }_1}{A_1}\\ \frac{{\beta }_2}{A_2}& 0\end{array}\right).$$

The corresponding characteristic equation has the following form:

$${\lambda }^2-\frac{{\beta }_2{\gamma }_1}{A_1{A}_2}=0,$$

from which ${\lambda }_{1,2}=\pm \sqrt{\frac{{\beta }_2{\gamma }_1}{A_1{A}_2}}$.

• (i) If ${\beta }_2{\gamma }_1<A_1{A}_2$, then $|{\lambda }_{1,2}|<1$, i.e., $E_0$ is locally asymptotically stable.

• (ii) If ${\beta }_2{\gamma }_1=A_1{A}_2$, then $|{\lambda }_{1,2}|=1$, which implies that $E_0$ is non-hyperbolic.

• (iii) If ${\beta }_2{\gamma }_1>A_1{A}_2$, then $|{\lambda }_{1,2}|>1$, which implies that $E_0$ is a repeller.

 □

Theorem 7

• (1) Assume that ${\beta }_2{\gamma }_1>A_1{A}_2$ and

  $${\beta }_2{\gamma }_1-A_1{A}_2>-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)].$$

  (8)

Then the positive equilibrium $E_{+}$ is a saddle point.

• (2) Assume that

  $$0<{\beta }_2{\gamma }_1-A_1{A}_2=-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)].$$

  (9)

Then the positive equilibrium $E_{+}$ is a non-hyperbolic point and

$$\overline{x}=-A_1+\sqrt{{\gamma }_1(A_1{B}_2-A_2)},\overline{y}=\frac{(-A_1+\sqrt{{\gamma }_1(A_1{B}_2-A_2)})\sqrt{{\gamma }_1(A_1{B}_2-A_2)}}{{\gamma }_1}.$$

• (3) Assume that

  $$0<{\beta }_2{\gamma }_1-A_1{A}_2<-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)].$$

  (10)

Then the positive equilibrium $E_{+}$ is locally asymptotically stable.

Proof The Jacobian matrix of T at the equilibrium $E_{+}=(\overline{x},\overline{y})$ is of the form (7) and the corresponding characteristic equation has the following form:

$${\lambda }^2-p\lambda +q=0,$$

where

Hence, for $E_{+}=(\overline{x},\overline{y})$, we have $p<0$, $q<0$, so $p^2-4q>0$. Since

$$\begin{array}{rcl}p-q-1& =& -\frac{{\overline{x}}^2}{{\gamma }_1\overline{y}}-\frac{{\overline{y}}^2}{{\beta }_2\overline{x}}-\frac{\overline{x}\overline{y}}{{\beta }_2{\gamma }_1}+\frac{\overline{y}(A_2+\overline{y})}{{\beta }_2\overline{x}}-1\stackrel{(\text{4})}{=}-\frac{{\overline{x}}^2}{{\gamma }_1\overline{y}}-\frac{{\overline{y}}^2}{{\beta }_2\overline{x}}-\frac{\overline{x}\overline{y}}{{\beta }_2{\gamma }_1}+(1-\frac{B_2\overline{y}}{{\beta }_2})-1\\ =& -\frac{{\overline{x}}^2}{{\gamma }_1\overline{y}}-\frac{{\overline{y}}^2}{{\beta }_2\overline{x}}-\frac{\overline{x}\overline{y}}{{\beta }_2{\gamma }_1}-\frac{B_2\overline{y}}{{\beta }_2}<0,\end{array}$$

we obtain

$$|p|\{\begin{array}{c}>|1+q|,\hfill \\ =|1+q|,\hfill \\ <|1+q|\hfill \end{array}\iff 1+p+q\{\begin{array}{c}<0,\hfill \\ =0,\hfill \\ >0.\hfill \end{array}$$

Similarly,

$$\begin{array}{rcl}1+p+q& =& 1-\frac{\overline{x}}{A_1+\overline{x}}-\frac{\overline{y}}{A_2+B_2\overline{x}+\overline{y}}+\frac{\overline{x}\overline{y}}{(A_1+\overline{x})(A_2+B_2\overline{x}+\overline{y})}-\frac{A_2+\overline{y}}{A_2+B_2\overline{x}+\overline{y}}\\ =& -\frac{A_2\overline{x}+\overline{y}(A_1+\overline{x})-A_1{B}_2\overline{x}}{(\overline{x}+A_1)(A_2+B_2\overline{x}+\overline{y})}\\ \stackrel{(\text{4})}{=}& -\frac{\overline{x}}{{\gamma }_1(\overline{x}+A_1)(A_2+B_2\overline{x}+\overline{y})}\varphi (\overline{x}),\end{array}$$

where

$$\varphi (x)=x^2+2A_{1}x+A_1^2+{\gamma }_1(A_2-A_1{B}_2),\text{for }x>0.$$

Now, for the positive equilibrium, it holds

If $A_1^2+{\gamma }_1(A_2-A_1{B}_2)\ge 0$, then $\varphi (x)>0$ for all $x>0$, which implies that $E_{+}$ is a saddle point. If $A_1^2+{\gamma }_1(A_2-A_1{B}_2)<0$, then $\varphi (x)=0$ for $x_{\pm }=-A_1\pm \sqrt{{\gamma }_1(A_1{B}_2-A_2)}$ ($x_{-}<0$, $x_{+}>0$).

Now we have three cases: $x_{+}<\overline{x}$, $x_{+}=\overline{x}$ or $\overline{x}<x_{+}$. Functions $f(x)$ and $\varphi (x)$ are increasing for $x>0$.

• (1) If $x_{+}<\overline{x}$, then $0=\varphi (x_{+})<\varphi (\overline{x})$, i.e., $1+p+q<0$ and $f(x_{+})<f(\overline{x})=0$. So,

  

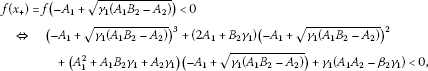
from which it follows

$${\gamma }_1{B}_2(A_1{B}_2-A_2)<({\beta }_2{\gamma }_1-A_1{A}_2)+A_1^2{B}_2,$$

i.e.,

$${\beta }_2{\gamma }_1>(A_1-{\gamma }_1{B}_2)(A_2-A_1{B}_2).$$

(11)

Now we have

$${\beta }_2{\gamma }_1-A_1{A}_2>-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)],$$

so we can see that the conditions (8) and (6) are sufficient for $E_{+}=(\overline{x},\overline{y})$ to be a saddle point.

• (2) If $x_{+}=\overline{x}$, then $0=\varphi (x_{+})=\varphi (\overline{x})$, hence $1+p+q=0$, i.e.,

  $$f(x_{+})=f(\overline{x})=f(-A_1+\sqrt{{\gamma }_1(A_1{B}_2-A_2)})=0,$$

from which

$${\beta }_2{\gamma }_1=(A_1-{\gamma }_1{B}_2)(A_2-A_1{B}_2).$$

(12)

If conditions (12) and (6) are satisfied, then

$${\beta }_2{\gamma }_2-A_1{A}_2=-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)]>0$$

holds, i.e., $E_{+}=(\overline{x},\overline{y})$ is a non-hyperbolic point of the form

$$\overline{x}=x_{+}=-A_1+\sqrt{{\gamma }_1(A_1{B}_2-A_2)},\overline{y}=\frac{(-A_1+\sqrt{{\gamma }_1(A_1{B}_2-A_2)})\sqrt{{\gamma }_1(A_1{B}_2-A_2)}}{{\gamma }_1}.$$

• (3) If $\overline{x}<x_{+}$, then $\varphi (\overline{x})<\varphi (x_{+})=0$ and

  $$0=f(\overline{x})<f(x_{+})=f(-A_1+\sqrt{{\gamma }_1(A_1{B}_2-A_2)}),$$

from which

$${\beta }_2{\gamma }_1<(A_1-{\gamma }_1{B}_2)(A_2-A_1{B}_2).$$

(13)

Hence, if conditions (13) and (6) are satisfied, then

$$0<{\beta }_2{\gamma }_2-A_1{A}_2<-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)]$$

holds, so $E_{+}$ is a locally asymptotically stable. □

4 Periodic character of solutions

In this section, we give the existence and local stability of period-two solutions.

Lemma 2 Assume that ${\beta }_2{\gamma }_1>A_1{A}_2$. Then System (2) has the following minimal period-two solutions:

$$A_0=(0,\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{{\gamma }_1{B}_2})\mathit{\text{and}}{B}_0=(\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{A_1{B}_2},0).$$

(14)

If

$$0<{\beta }_2{\gamma }_1-A_1{A}_2=-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)],$$

then System (2) has an infinite number of minimal period-two solutions of the form

for $x\in [0,\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{A_1{B}_2}]$, located along the line

$$\mathcal{H}=\{(x,y):x{A}_1+{\gamma }_{1}y+A_1^2+{\gamma }_1(A_2-A_1{B}_2)=0,x\in [0,\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{A_1{B}_2}]\}.$$

(15)

Proof The second iterate of T is (25). Equilibrium curves of the map $T^2(x,y)$ are

$$C_{1T^2}=\{(x,y)\in [0,\mathrm{\infty }{)}^2:x{\beta }_2{\gamma }_1(x+A_1)=x(y+A_2+x{B}_2)(A_1^2+x{A}_1+y{\gamma }_1)\}$$

(16)

and

$$\begin{array}{rcl}{C}_{2T^2}& =& \{(x,y)\in [0,\mathrm{\infty }{)}^2:y{\beta }_2{\gamma }_1(y+A_2+x{B}_2)=y(A_1{A}_2^2+x^2{\beta }_2+x{A}_2^2+x^2{A}_2{B}_2+xy{A}_2\\ +x{\beta }_2{A}_1+y{A}_1{A}_2+y^2{\gamma }_1{B}_2+y{\gamma }_1{A}_2{B}_2+x{A}_1{A}_2{B}_2+xy{\gamma }_1{B}_2^2\left)\right\}.\end{array}$$

(17)

We get period-two solutions as the intersection point of equilibrium curves (16) and (17) in the first quadrant. If $x\ne 0$, $y=0$, then System (16), (17) is reduced to the equation

$${\beta }_2{\gamma }_1(x+A_1)=A_1(A_2+x{B}_2)(x+A_1),$$

and the positive solution of this equation is

$$x=\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{A_1{B}_2}>0,\text{for }{\beta }_2{\gamma }_1-A_1{A}_2>0.$$

If $x=0$, $y\ne 0$, then System (16), (17) is reduced to the equation

$${\beta }_2{\gamma }_1(y+A_2)=(y+A_2)(A_1{A}_2+y{\gamma }_1{B}_2),$$

with the positive solution

$$y=\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{{\gamma }_1{B}_2}>0,\text{for }{\beta }_2{\gamma }_1-A_1{A}_2>0.$$

On the other hand, if $x>0$, $y>0$, then we have

$$\begin{array}{l}{\beta }_2{\gamma }_1(x+A_1)=(y+A_2+x{B}_2)(A_1^2+x{A}_1+y{\gamma }_1)\\ {\beta }_2{\gamma }_1(y+A_2+x{B}_2)=A_1{A}_2^2+x^2{\beta }_2+x{A}_2^2+x^2{A}_2{B}_2+xy{A}_2+x{\beta }_2{A}_1+y{A}_1{A}_2\\ \phantom{{\beta }_2{\gamma }_1(y+A_2+x{B}_2)=}+y^2{\gamma }_1{B}_2+y{\gamma }_1{A}_2{B}_2+x{A}_1{A}_2{B}_2+xy{\gamma }_1{B}_2^2\end{array}\},$$

that is

$$(x+A_1)({\beta }_2{\gamma }_1-A_1{A}_2)=(y+x{B}_2)(A_1^2+x{A}_1+y{\gamma }_1)+y{\gamma }_1{A}_2$$

(18)

and

(19)

Therefore, it must be $({\beta }_2{\gamma }_1-A_1{A}_2)>0$ in order to get any positive solution. By eliminating the term $({\beta }_2{\gamma }_1-A_1{A}_2)$ from (18) and using condition (9), we get

$$(y+x{B}_2+A_1{B}_2)(y{\gamma }_1+x{A}_1+A_1^2+{\gamma }_1{A}_2-{\gamma }_1{A}_1{B}_2)=0,$$

which implies

$$y{\gamma }_1+x{A}_1+A_1^2+{\gamma }_1(A_2-A_1{B}_2)=0,$$

hence

$$y=-\frac{1}{{\gamma }_1}(x{A}_1+A_1^2+{\gamma }_1(A_2-A_1{B}_2)),{\gamma }_1\ne 0.$$

(20)

Now, by eliminating y and the term $(A_1{A}_2-{\beta }_2{\gamma }_1)$ from (19), we get the identity

$$(x+A_1)(x+A_1-{\gamma }_1{B}_2)\frac{{\beta }_2{\gamma }_1-(A_2-A_1{B}_2)(A_1-{\gamma }_1{B}_2)}{{\gamma }_1}=0.$$

If $x={\gamma }_1{B}_2-A_1$, we have

$$y=-\frac{1}{{\gamma }_1}(x{A}_1+A_1^2+{\gamma }_1(A_2-A_1{B}_2))=-A_2<0,{\gamma }_1\ne 0.$$

So, periodic solutions are located along line (15) with endpoints given by (14) using conditions (9). It is easy to see that $A_x,B_x\in \mathcal{H}$ if ${\beta }_2{\gamma }_1-A_1{A}_2=-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)]$. □

Let $(x,y)\in \mathcal{H}$, then the corresponding Jacobian matrix of the map $T^2$ has the following form:

$$J_{T^2}^{\mathcal{H}}(x,y)=\left(\begin{array}{cc}a& b\\ c& d\end{array}\right),$$

(21)

where $a:=F_x(x,y)$, $b:=F_y(x,y)$, $c:=G_x(x,y)$, $d:=G_y(x,y)$.

Lemma 3 Assume that $0<{\beta }_2{\gamma }_1-A_1{A}_2=-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)]$. Then the following statements are true.

• (a) The points $A_x,B_x\in \mathcal{H}$ are non-hyperbolic fixed points for the map $T^2$, and both of them have eigenvalues ${\lambda }_1=1$ and ${\lambda }_2\in (0,1)$.

• (b) Eigenvectors corresponding to the eigenvalues ${\lambda }_1$ and ${\lambda }_2$ are not parallel to coordinate axes.

Proof (a) From (15) we have $y_{\mathcal{H}}^{\mathrm{\prime }}(x)=-\frac{A_1}{{\gamma }_1}<0$. Since

$$\mathcal{H}=\{(x,y)\in [0,\mathrm{\infty }{)}^2:F(x,y)=x\}=\{(x,y)\in [0,\mathrm{\infty }{)}^2:G(x,y)=y\},$$

by implicit differentiation of equations $F(x,y)=x$ and $G(x,y)=y$ at the point $(x,y)\in \mathcal{H}$, we obtain

$$y_{\mathcal{H}}^{\mathrm{\prime }}(x)=\frac{1-a}{b}=\frac{c}{1-d}=-\frac{A_1}{{\gamma }_1}<0.$$

(22)

Since $a>0$, $b<0$, $c<0$ and $d>0$, from (22), we get

$$0<a<1\text{and}0<d<1.$$

(23)

The characteristic polynomial of the matrix (21) at the point $(x,y)\in \mathcal{H}$ is of the form

$$P(\lambda )={\lambda }^2-(a+d)\lambda +(ad-bc).$$

Now, using (22) we have $(1-a)(1-d)=bc$, and since

$$P(1)=1-(a+d)+(ad-bc)=0,$$

we get ${\lambda }_1=1$, and due to Vieta’s formulas and condition (23), it follows

$$0<{\lambda }_1+{\lambda }_2=1+{\lambda }_2=a+d<2,$$

i.e., $0<{\lambda }_2<1$.

(b) Eigenvectors corresponding to the eigenvalues ${\lambda }_1$ and ${\lambda }_2$ are ${\mathbf{v}}_1=(1-d,c)$ and ${\mathbf{v}}_2=(a-1,c)$. By condition (23) it is easy to see that these vectors are not parallel to the coordinate axes. □

Lemma 4 The periodic points $A_0$ and $B_0$ given by (14) are

• (a) locally asymptotically stable if ${\beta }_2{\gamma }_1-A_1{A}_2>-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)]$ and ${\beta }_2{\gamma }_1>A_1{A}_2$,

• (b) non-hyperbolic if $0<{\beta }_2{\gamma }_1-A_1{A}_2=-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)]$,

• (c) saddle points if $0<{\beta }_2{\gamma }_1-A_1{A}_2<-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)]$.

Proof We have that

$$J_{T^2}(\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{A_1{B}_2},0)=\left(\begin{array}{cc}\frac{A_1{A}_2}{{\beta }_2{\gamma }_1}& \frac{({\beta }_2{\gamma }_1-A_1{A}_2)(A_1^2{A}_2-A_1^3{B}_2-{\beta }_2{\gamma }_1^2{B}_2-{\beta }_2{\gamma }_1{A}_1)}{{\beta }_2{\gamma }_1{A}_1{B}_2(A_1^2{B}_2+{\beta }_2{\gamma }_1-A_1{A}_2)}\\ 0& \frac{{\beta }_2{\gamma }_1^2{A}_1{B}_2^2}{(A_1^2{B}_2+{\beta }_2{\gamma }_1-A_1{A}_2)({\beta }_2{\gamma }_1-A_1{A}_2+{\gamma }_1{A}_2{B}_2)}\end{array}\right)$$

and characteristic eigenvalues are

$${\lambda }_1=\frac{A_1{A}_2}{{\beta }_2{\gamma }_1}<1\text{and}{\lambda }_2=\frac{{\beta }_2{\gamma }_1^2{A}_1{B}_2^2}{({\beta }_2{\gamma }_1-A_1{A}_2+{\gamma }_1{A}_2{B}_2)(B_2{A}_1^2-A_2{A}_1+{\beta }_2{\gamma }_1)}.$$

Now,

Therefore,

On the other hand, we have

$$J_{T^2}(0,\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{{\gamma }_1{B}_2})=\left(\begin{array}{cc}\frac{{\beta }_2{\gamma }_1^2{A}_1{B}_2^2}{(A_1^2{B}_2+{\beta }_2{\gamma }_1-A_1{A}_2)({\beta }_2{\gamma }_1-A_1{A}_2+{\gamma }_1{A}_2{B}_2)}& 0\\ \frac{({\beta }_2{\gamma }_1-A_1{A}_2)(A_1{A}_2^2-{\gamma }_1{A}_2^2{B}_2-{\beta }_2{\gamma }_1{A}_2-{\beta }_2{\gamma }_1{A}_1{B}_2)}{{\beta }_2{\gamma }_1^2{B}_2({\beta }_2{\gamma }_1-A_1{A}_2+{\gamma }_1{A}_2{B}_2)}& \frac{A_1{A}_2}{{\beta }_2{\gamma }_1}\end{array}\right)$$

and the corresponding eigenvalues are

$${\lambda }_1=\frac{A_1{A}_2}{{\beta }_2{\gamma }_1}<1\text{and}{\lambda }_2=\frac{{\beta }_2{\gamma }_1^2{A}_1{B}_2^2}{({\beta }_2{\gamma }_1-A_1{A}_2+{\gamma }_1{A}_2{B}_2)(B_2{A}_1^2-A_2{A}_1+{\beta }_2{\gamma }_1)},$$

so it comes to the same conclusion! □

5 Global results

In this section, we present the results on the global dynamics of System (2).

Lemma 5 Every solution of System (2) satisfies

• 1. $x_n\le \frac{{\gamma }_1}{A_1}\cdot \frac{{\beta }_2}{B_2}$, $y_n\le \frac{{\beta }_2}{B_2}$, $n=2,3,\dots$.

• 2. If ${\beta }_2{\gamma }_1<A_1{A}_2$, then ${lim}_{n\to \mathrm{\infty }}{x}_n=0$, ${lim}_{n\to \mathrm{\infty }}{y}_n=0$.

The map T satisfies:

• 3. $T(\mathcal{B})\subseteq \mathcal{B}$, where $\mathcal{B}=[0,\frac{{\gamma }_1}{A_1}\cdot \frac{{\beta }_2}{B_2}]\times [0,\frac{{\beta }_2}{B_2}]$, that is, $\mathcal{B}$ is an invariant box.

• 4. $T(\mathcal{B})$ is an attracting box, that is $T{([0,\mathrm{\infty })}^2)\subseteq \mathcal{B}$.

Proof From System (2), we have

for $n=0,1,2,\dots$ , and

$$x_{n+1}\le \frac{{\gamma }_1}{A_1}{y}_n\le \frac{{\gamma }_1}{A_1}\cdot \frac{{\beta }_2}{B_2}$$

for $n=1,2,\dots$ . Furthermore, we get

$$x_n\le \frac{{\gamma }_1}{A_1}{y}_{n-1}\le \frac{{\gamma }_1{\beta }_2}{A_1{A}_2}{x}_{n-2},$$

i.e.,

$$x_{2n}\le {\left(\frac{{\gamma }_1{\beta }_2}{A_1{A}_2}\right)}^n{x}_0,x_{2n+1}\le {\left(\frac{{\gamma }_1{\beta }_2}{A_1{A}_2}\right)}^n{x}_1,$$

so it follows that ${lim}_{n\to \mathrm{\infty }}{x}_n=0$, ${lim}_{n\to \mathrm{\infty }}{y}_n=0$ if ${\beta }_2{\gamma }_1<A_1{A}_2$.

Proof of 3. and 4. is an immediate checking. □

Lemma 6 The map $T^2$ is injective and $det{J}_{T^2}(x,y)>0$, for all $x\ge 0$ and $y\ge 0$.

Proof (i) Here we prove that map T is injective, which implies that $T^2$ is injective. Indeed, $T\left(\begin{array}{c}{x}_1\\ y_1\end{array}\right)=T\left(\begin{array}{c}{x}_2\\ y_2\end{array}\right)$ implies that

$$A_1(y_1-y_2)=x_1{y}_2-x_2{y}_1,A_2(x_1-x_2)=x_2{y}_1-x_1{y}_2.$$

(24)

By solving System (24) with respect to $x_1$, $x_2$ or $y_1$, $y_2$, we obtain that $(x_1,y_1)=(x_2,y_2)$.

• (ii) The map $T^2(x,y)=\left(\begin{array}{c}F(x,y)\\ G(x,y)\end{array}\right)$ is of the form

  

  (25)

and

$$J_{T^2}(x,y)=\left(\begin{array}{cc}{F}_x& F_y\\ G_x& G_y\end{array}\right),$$

where

Now, we obtain

$$det{J}_{T^2}(x,y)=F_x{G}_y-F_y{G}_x=UV,$$

where

and the Jacobian matrix of $T^2(x,y)$ is invertible for all $x\ge 0$ and $y\ge 0$. □

Corollary 1 The competitive map $T^2$ satisfies the condition (O+). Consequently, the sequences $\{x_{2n}\}$, $\{x_{2n+1}\}$, $\{y_{2n}\}$, $\{y_{2n+1}\}$ of every solution of System (2) are eventually monotone.

Proof It immediately follows from Lemma 6, Theorem 2 and 3. □

Lemma 7 Assume ${\beta }_2{\gamma }_1-A_1{A}_2>0$. System (2) has period-two solutions (14) and

• (a) If $(x_0,y_0)=(x,0)$, $x>0$, then

  $$\underset{n\to \mathrm{\infty }}{lim}{T}^{2n}(x,0)=(\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{A_1{B}_2},0)=B_0$$

and

$$\underset{n\to \mathrm{\infty }}{lim}{T}^{2n+1}(x,0)=(0,\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{{\gamma }_1{B}_2})=A_0.$$

• (b) If $(x_0,y_0)=(0,y)$, $y>0$, then

  $$\underset{n\to \mathrm{\infty }}{lim}{T}^{2n}(0,y)=(0,\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{{\gamma }_1{B}_2})=A_0$$

and

$$\underset{n\to \mathrm{\infty }}{lim}{T}^{2n+1}(x,0)=(\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{A_1{B}_2},0)=B_0.$$

Proof (a) For all $x>0$, $x\ne \frac{{\beta }_2{\gamma }_1-A_1{A}_2}{A_1{B}_2}$, we have

and by induction,

Now, we have

and

 □

Lemma 8 The map $T^2$ associated to System (2) satisfies the following:

$$T^2(x,y)=(\overline{x},\overline{y})\mathit{\text{only for}}(x,y)=(\overline{x},\overline{y}).$$

Proof Since $T^2$ is injective, then $T^2(x,y)=(\overline{x},\overline{y})=T^2(\overline{x},\overline{y})\Rightarrow (x,y)=(\overline{x},\overline{y})$. □

Proof of Theorem 1 Case 1 ${\beta }_2{\gamma }_1\le A_1{A}_2$

Equilibrium $E_0$ is unique (see Lemma 1), and by Lemma 5, every solution of System (2) belongs to

$$B=\left[\begin{array}{c}0,\frac{{\beta }_2{\gamma }_1}{A_1{B}_2}\end{array}\right]\times \left[\begin{array}{c}0,\frac{{\beta }_2}{B_2}\end{array}\right],$$

which is an invariant box. In view of Corollary 1 and Theorem 2, every solution converges to minimal period-two solutions or $E_0$. System (2) has no minimal period-two solutions (Lemma 2). So, every solution of System (2) converges to $E_0$.

Case 2 ${\beta }_2{\gamma }_1-A_1{A}_2>-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)]$ and ${\beta }_2{\gamma }_1-A_1{A}_2>0$

By Lemmas 1, 2, 4 and Theorems 6 and 7, there exist two equilibrium points: $E_0$ which is a repeller and $E_{+}$ which is a saddle point, and minimal period-two solutions $A_0$ and $B_0$ which are locally asymptotically stable. Clearly $T^2$ is strongly competitive and it is easy to check that the points $A_0$ and $B_0$ are locally asymptotically stable for $T^2$ as well. System (2) can be decomposed into the system of the even-indexed and odd-indexed terms as follows:

$$\{\begin{array}{c}{x}_{2n+1}=\frac{{\gamma }_1{y}_{2n}}{A_1+x_{2n}},\hfill \\ x_{2n}=\frac{{\gamma }_1{y}_{2n-1}}{A_1+x_{2n-1}},\hfill \\ y_{2n+1}=\frac{{\beta }_2{x}_{2n}}{A_2+B_2{x}_{2n}+y_{2n}},\hfill \\ y_{2n}=\frac{{\beta }_2{x}_{2n-1}}{A_2+B_2{x}_{2n-1}+y_{2n-1}},n=1,2,\dots .\hfill \end{array}$$

The existence of the set $\mathcal{C}$ with the stated properties follows from Lemmas 6, 2, 7, 8, Corollary 1, Theorems 4 and 5.

Case 3 $0<{\beta }_2{\gamma }_1-A_1{A}_2=-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)]$

Cases (i) and (ii) from (c) in Theorem 1 are the consequence of Lemmas 1, 2, 4 and Theorems 6 and 7.

Since $T^2$ is strongly competitive and points $A_x$ and $B_x$, for all $x\in [0,\frac{{\beta }_2{\gamma }_1-A_1{A}_2}{A_1{B}_2})$, are non-hyperbolic points of the map $T^2$, by Lemmas 1, 6, 2, 3, 7, Corollary 1, Theorems 2, 5, 6 and 7, it follows that all conditions of Theorem 4 are satisfied for the map $T^2$ with $\mathcal{R}=[0,\mathrm{\infty })\times [0,\mathrm{\infty })$. By Lemma 7, it is clear that

$${\mathcal{C}}_{A_0}=\{(x,y):x=0,y>0\}\text{and}{\mathcal{C}}_{B_0}=\{(x,y):x>0,y=0\}.$$

Case 4 $0<{\beta }_2{\gamma }_1-A_1{A}_2<-B_2[A_1^2+{\gamma }_1(A_2-A_1{B}_2)]$

Lemma 2 implies that System (2) has minimal period-two solutions (14). Furthermore, Corollary 1 and Theorem 2 imply that all solutions of System (2) converge to an equilibrium or minimal period-two solutions, and since, by Theorem 6, $E_0$ is a repeller, all solutions converge to $E_{+}$ (which is, in view of Theorem 7, locally asymptotically stable) or minimal period-two solutions (14). The points $A_0$ and $B_0$ are saddle points of the strongly competitive map $T^2$; and by Lemma 7, the stable manifold of $A_0$ (under $T^2$) is

$$\mathcal{B}(A_0)=\{(x,y):x=0,y>0\}$$

and the stable manifold of $B_0$ (under $T^2$) is

$$\mathcal{B}(B_0)=\{(x,y):x>0,y=0\}$$

and each of these stable manifolds is unique. This implies that the basin of attraction of the equilibrium point $E_{+}$ is the set

$$\mathcal{B}(E_{+})=\{(x,y):x>0,y>0\},$$

and Lemma 7 completes the conclusion (d) of Theorem 1. □