- Research Article
- Open access
- Published:
Results and Conjectures about Order 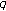 Lyness' Difference Equation
Lyness' Difference Equation 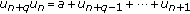 in
in 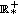 , with a Particular Study of the Case
, with a Particular Study of the Case 
Advances in Difference Equations volume 2009, Article number: 134749 (2009)
Abstract
We study order  Lyness' difference equation in
Lyness' difference equation in  , with
, with  and the associated dynamical system
and the associated dynamical system  in
in  . We study its solutions (divergence, permanency, local stability of the equilibrium). We prove some results, about the first three invariant functions and the topological nature of the corresponding invariant sets, about the differential at the equilibrium, about the role of 2-periodic points when
. We study its solutions (divergence, permanency, local stability of the equilibrium). We prove some results, about the first three invariant functions and the topological nature of the corresponding invariant sets, about the differential at the equilibrium, about the role of 2-periodic points when  is odd, about the nonexistence of some minimal periods, and so forth
is odd, about the nonexistence of some minimal periods, and so forth and discuss some problems, related to the search of common period to all solutions, or to the second and third invariants. We look at the case
and discuss some problems, related to the search of common period to all solutions, or to the second and third invariants. We look at the case  with new methods using new invariants for the map
with new methods using new invariants for the map  and state some conjectures on the associated dynamical system in
and state some conjectures on the associated dynamical system in  in more general cases.
in more general cases.
Publisher note
To access the full article, please see PDF
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Bastien, G., Rogalski, M. Results and Conjectures about Order 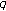 Lyness' Difference Equation
Lyness' Difference Equation 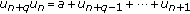 in
in 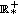 , with a Particular Study of the Case
, with a Particular Study of the Case  .
Adv Differ Equ 2009, 134749 (2009). https://doi.org/10.1155/2009/134749
.
Adv Differ Equ 2009, 134749 (2009). https://doi.org/10.1155/2009/134749
Received:
Accepted:
Published:
DOI: https://doi.org/10.1155/2009/134749